What Are Consecutive Interior Angles? Theorems, Proofs, and Examples

Everything you need to know to ace a test on Consecutive Interior Angles

Co-authored by Johnathan Fuentes

Last Updated: February 6, 2023 References

What are consecutive interior angles?

|

Consecutive Interior Angle Theorem

|

Consecutive Interior Angle Theorem Proof

|

Converse of the Consecutive Interior Angle Theorem

|

Converse Consecutive Interior Angle Theorem Proof

|

Examples Using the Consecutive Interior Angle Theorem

Got a geometry test coming up? Need help with a homework assignment on consecutive interior angles? Look no further. We’ve created a total guide to Consecutive Interior Angles, complete with explanations and examples to boost your understanding. We’ll also explain the Consecutive Interior Angle Theorem—and the Converse Consecutive Interior Angle Theorem—and provide proofs for both. Keep reading to learn what consecutive interior angles are, how they work, and what to do when you encounter them on a test or quiz.

Things You Should Know

Consecutive interior angles are pairs of angles on one side of a line—called a “transversal”—that crosses two other lines.
The Consecutive Interior Angle Theorem states that when the two lines crossed by the transversal are parallel, the consecutive interior angles add up to 180°.^{[1]
X
Research source}
The Converse Consecutive Interior Angle Theorem states that when a transversal intersects two lines and each pair of consecutive interior angles adds up to 180°, the two lines must be parallel.

Section 1 of 6:

What are consecutive interior angles?

Consecutive interior angles are the pairs of angles on one side of a transversal, in between two lines. A transversal is a line that crosses two other lines.^{[2]
X
Research source} In the image above, the transversal is highlighted in orange, while the lines it crosses are black.^{[3]
X
Research source}^{[4]
X
Research source}
- The red angles, ∠C & ∠E, are on one side of the transversal, in between the two black lines. Therefore, ∠C & ∠E are consecutive interior angles.
- The blue angles, ∠D & ∠F, are also on one side of the transversal (in this case, on the other side), in between the two lines. Therefore, ∠D & ∠F are consecutive interior angles.
- ∠A, ∠B, ∠G, and ∠H are not in between the two lines. Therefore, they are not consecutive interior angles.

Section 2 of 6:

Consecutive Interior Angle Theorem

This theorem states that when a transversal intersects two parallel lines, each pair of consecutive interior angles are supplementary. In other words, as long as the two lines being intersected by the transversal are parallel, each of the resulting consecutive interior angle pairs adds up to 180°. To see how this works, let’s look at the example above.^{[5]
X
Research source}
- We see two parallel lines crossed by a transversal. Angles of the same value have matching colors. The purple angles = 150°, while the pink angles = 30°.
- Look closely at the two consecutive interior angles on the left side of the transversal. The top one (in pink) is 30°, while the bottom one (in purple) is 150°. By adding them, we see that 30°+ 150° = 180°.
- Likewise, the consecutive interior angles on the right side of the transversal are 150° and 30°, which add up to 180°.

Section 3 of 6:

Consecutive Interior Angle Theorem Proof

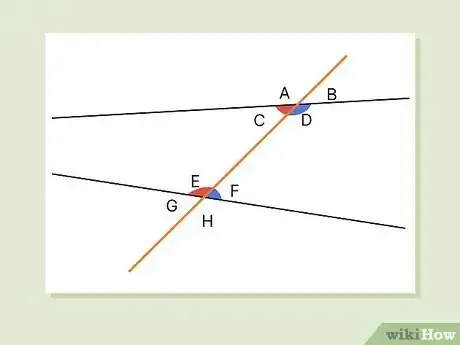
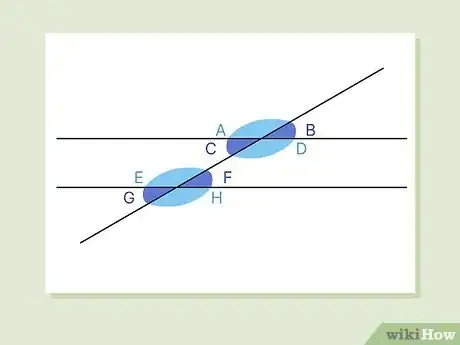
1
Look closely at the figure above. There are two parallel lines crossed by a transversal. We can see that the two pairs of consecutive interior angles are ∠C & ∠E and ∠D & ∠F. However, we don’t know the values of the angles.
- How do we prove that each pair of consecutive interior angles adds up to 180°, without knowing what those angles are?
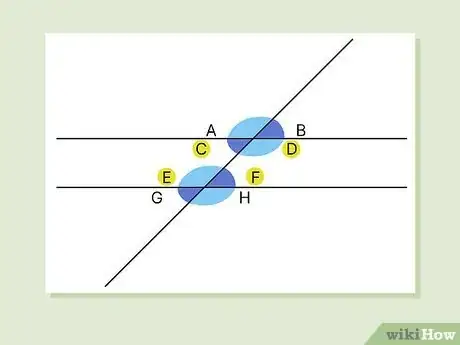
2
Look at ∠A & ∠B. Since they come together to form a straight line, we know that they add up to 180°. The same is true for ∠C & ∠D, ∠E & ∠F, and ∠G & ∠H. We also know from geometry that two intersecting lines produce identical angles on opposite sides.^{[6]
X
Research source}
- This means that ∠A = ∠D, and ∠B = ∠C.
- Likewise, ∠E = ∠H, and ∠F = ∠G.
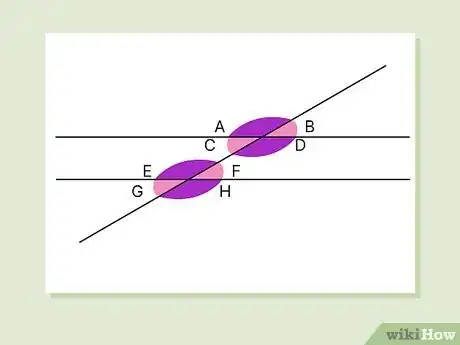
3
We know that the transversal intersects both lines at the same angles. This means that the angles on the top line are replicated on the bottom line.^{[7]
X
Research source} In other words:
- ∠A = ∠E
- ∠B = ∠F
- ∠C = ∠G
- ∠D = ∠H
4
We now have all the information we need to prove the theorem. Since ∠A = ∠D, and ∠B = ∠F, then ∠A + ∠B = ∠D + ∠F. Likewise, since ∠C = ∠G, and ∠E = ∠H, we know that ∠C + ∠E = ∠G + ∠H.
- Since ∠A + ∠B = 180°, ∠D + ∠F = 180°.
- Since ∠G + ∠H = 180°, ∠C + ∠E = 180° as well.
- This proves that both pairs of consecutive interior angles add up to 180°.

Section 4 of 6:

Converse of the Consecutive Interior Angle Theorem

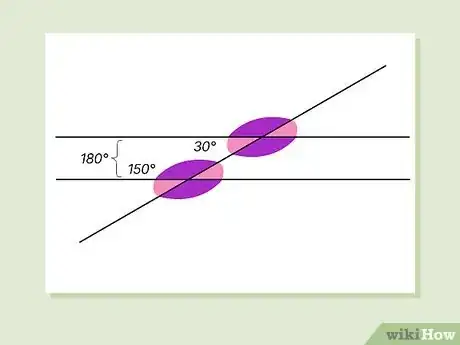
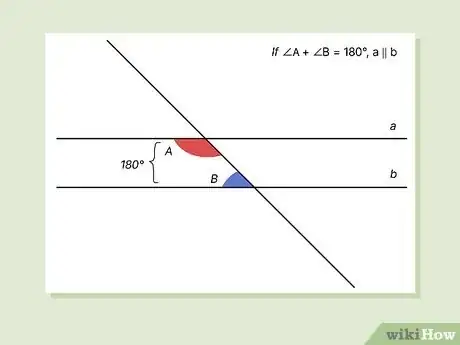
1
This theorem says that when a transversal intersects two lines so that each pair of consecutive interior angles adds up to 180°, the lines must be parallel. To illustrate this, let’s examine the figure above.^{[8]
X
Research source}
2
Look closely at the angles in this figure. You’ll that there are two pairs of angles, colored blue and red. The blue angles = 45°, while the red angles = 135°. Note the two pairs of consecutive interior angles. The top pair (located on the same side of the transversal) are 135° and 45°. The bottom pair are 45° and 135°.
- Since 45° + 135° = 180°, both pairs of consecutive interior angles add up to 180°.
- We know that consecutive interior angles only add up to 180° when the transversal intersects parallel lines.
- Therefore, since our consecutive interior angles add up to 180°, we know that the lines must be parallel.

Section 5 of 6:

Converse Consecutive Interior Angle Theorem Proof

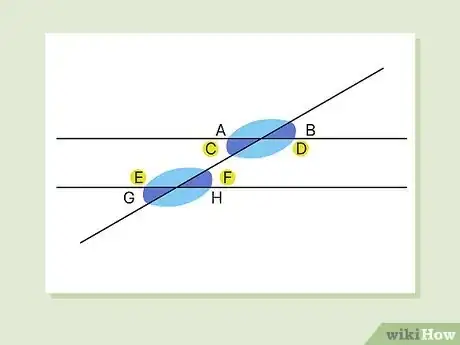
1
Examine the figure above. We see two lines crossed by a transversal, but we’re not sure if the lines are parallel. However, we know that ∠A = ∠E, ∠B = ∠F, ∠C = ∠G, and ∠D = ∠H. Note the two pairs of consecutive interior angles: ∠C & ∠E, and ∠D & ∠F.
- How can we prove that the lines are parallel, without knowing the exact values of the angles?
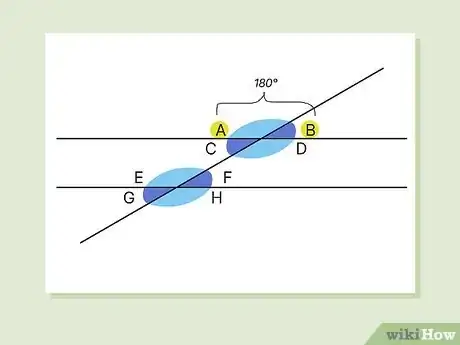
2
Next, let’s prove that the consecutive interior angles add up to 180°. We can see that ∠A + ∠B = 180°. We know that ∠B = ∠C, since intersecting lines create identical angles on opposite sides. Since ∠A = ∠E, we also know that ∠A + ∠B = ∠E + ∠C. Therefore, since ∠A + ∠B = 180°, ∠E + ∠C = 180° as well. We can use the same process to show that ∠D + ∠F = 180°.
- We know that consecutive interior angles only add up to 180° when a transversal crosses parallel lines.
- Therefore we can deduce that the lines in the example above must be parallel.

Section 6 of 6:

Examples Using the Consecutive Interior Angle Theorem

1
Example 1: Assume that the transversal crosses two parallel lines. The consecutive interior angles are ∠C & ∠E, and ∠D & ∠F. What information do we know just from looking at the diagram? How can we use this information to prove that the consecutive interior angles are supplementary—that is, that they add up to 180°?
- We know that ∠A + ∠B = 180°, since they come together to form a line. The same is true for ∠C + ∠D, ∠E + ∠F, and ∠G + ∠H.^{[9]
  X
  Research source}
- We also know that ∠A = ∠D, ∠B = ∠C, ∠E = ∠H, and ∠F = ∠G, since intersecting lines produce identical angles on opposite sides.
- Lastly, we know that the angles on the top line are replicated on the bottom line, since a transversal always intersects parallel lines at the same angles. Therefore ∠A = ∠E, ∠B = ∠F, ∠C = ∠G, and ∠D = ∠H.
- Since ∠A = ∠E and ∠B = ∠C, we know that ∠A + ∠B = ∠E + ∠C. Since ∠A + ∠B = 180°, ∠E + ∠C = 180° as well. We can use the same process to show that ∠D + ∠F = 180°. Therefore, the consecutive interior angles are supplementary.
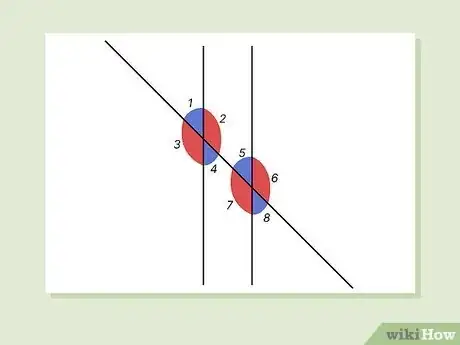
2
Example 2: We know the transversal crosses two parallel lines, and the consecutive interior angles are ∠2 & ∠4, and ∠5 & ∠7. What else do we know from looking at the diagram? How can we use this information to prove that the consecutive interior angles are supplementary?
- ∠1 + ∠3 = 180°, since they come together to form a line. ∠2 + ∠4, ∠5 + ∠7, and ∠6 + ∠8 = 180° as well.^{[10]
  X
  Research source}
- We know that ∠1 = ∠4, ∠2 = ∠3, ∠5 = ∠8, and ∠6 = ∠7, since intersecting lines produce identical angles on opposite sides.
- We also know that the angles on the left line are replicated on the right line, since a transversal always intersects parallel lines at the same angles. Thus ∠1 = ∠5, ∠2 = ∠6, ∠3 = ∠7, and ∠4 = ∠8.
- Since ∠1 = ∠5 and ∠2 = ∠3, we know that ∠1 + ∠3 = ∠5 + ∠2. Since ∠1 + ∠3 = 180°, ∠5 + ∠2 = 180°. The same process shows that ∠4 + ∠7 = 180°. Therefore, the consecutive interior angles are supplementary.
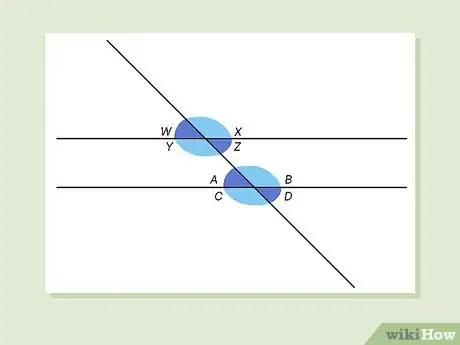
3
Example 3: Examine the figure above. Assume that the lines crossed by the transversal are parallel. Which angles form pairs of consecutive interior angles, and how can we prove that they’re supplementary?
- The consecutive interior angles are ∠Y & ∠A and ∠Z & ∠B.
- ∠W + ∠X = 180°, since they come together to form a line. ∠Y + ∠Z, ∠A + ∠B, and ∠C + ∠D = 180° as well.
- Since intersecting lines produce identical angles on opposite sides, we know that ∠W = ∠Z, ∠X = ∠Y, ∠A = ∠D, and ∠B = ∠C.
- We also know that the angles on the top line are replicated on the bottom line, since a transversal always intersects parallel lines at the same angles. Thus ∠W = ∠A, ∠X = ∠B, ∠Y = ∠C, and ∠Z = ∠D.
- Since ∠W = ∠Z and ∠X = ∠B, we know that ∠W + ∠X = ∠Z + ∠B. Since ∠W + ∠X = 180°, ∠Z + ∠B = 180°. The same process shows that ∠Y + ∠A = 180°. Therefore, the consecutive interior angles are supplementary.