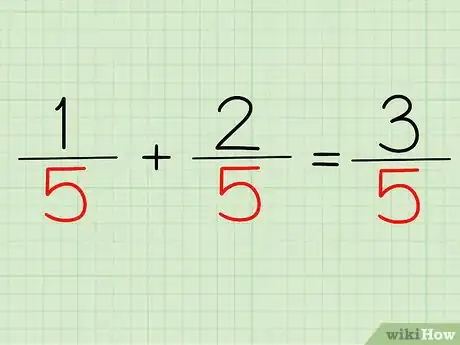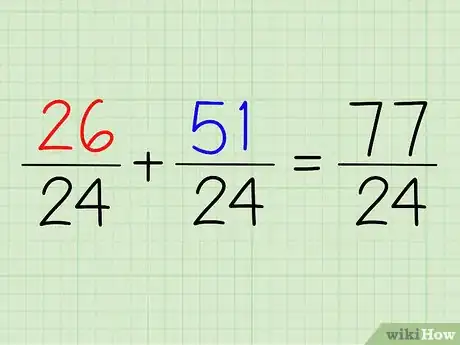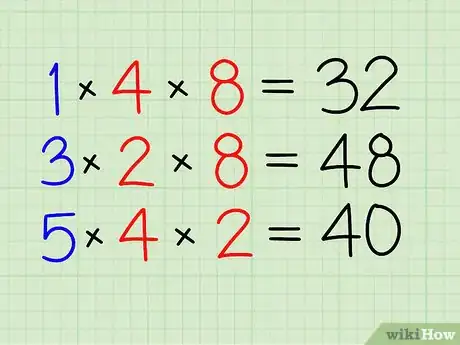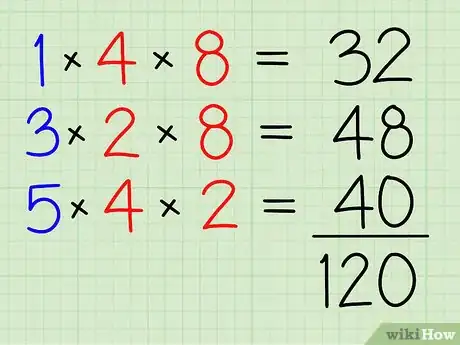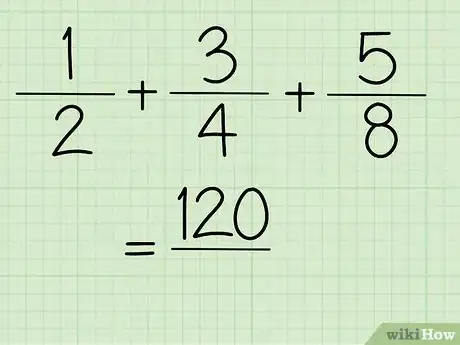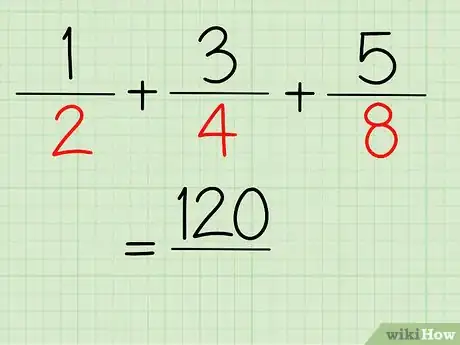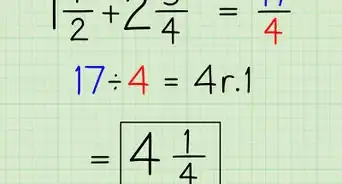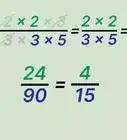How to Add and Subtract Fractions

Co-authored by David Jia

Last Updated: March 7, 2023 References

Adding and subtracting fractions is an essential skill to have. Fractions show up in daily life all the time, especially in math classes, from elementary school through college. Just follow these steps to learn how to add and subtract them, whether they're like fractions, unlike fractions, mixed, or improper fractions. Once you know one way, the rest is pretty easy!

Method 1

Method 1 of 4:

Adding and Subtracting Fractions with the Same Denominator

1
Write out your equation. If the denominator of the two fractions that you are adding or subtracting is the same, put the same number once as the denominator for your answer.^{[1]
X
Research source}
- In other words, 1/5 and 2/5 does not need to be written as 1/5 + 2/5 = ? It can be written as 1+2/5 = ?. The denominator is the same, so it can be written only once. Both numerators then go on top.
2
Add the numerators together. The "numerator" is the top number of any fraction.^{[2]
X
Research source} If we take the above example, 1/5 and 2/5, 1 and 2 are our numerators.
- Whether you have it written 1/5 + 2/5 or 1+2/5, you answer should be the same: 3! After all, 1 + 2 = 3.
Advertisement
3
Leave the denominator alone. Since you're working with one constant denominator, don't do anything with it! Don't add, subtract, multiply, or divide. Just leave it be.^{[3]
X
Research source}
- So, using the same example, our denominator is 5. That's it! That's the bottom number of our fraction. That's half the answer already!
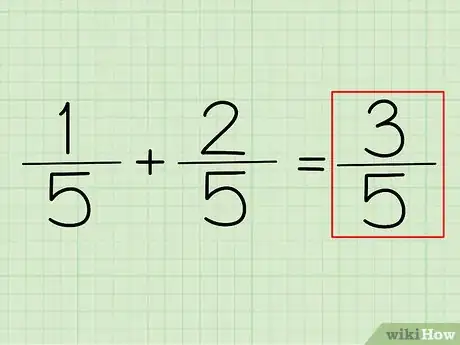
4
Come up with your answer. Now, all you do is write out your numerator and your denominator! If you've followed the above example, you'll find that the answer to this problem is 3/5.^{[4]
X
Research source}
- What was your numerator? 3. The denominator? 5. Therefore, 1/5 + 2/5, or 1+2/5, equals 3/5.

Method 2

Method 2 of 4:

Adding and Subtracting Fractions with Different Denominators

1
Find the lowest common denominator. This means the lowest number both denominators have in common.^{[5]
X
Research source} Let's take the fractions 2/3 and 3/4. What are the denominators? 3 and 4. To find the lowest common denominator of the two, you can do this one of three ways:
- Write out the multiples. The multiples of 3 are 3, 6, 9, 12, 15, 18...and so on. The multiples of 4? 4, 8, 12, 16, 20, etc. What's the lowest number seen in both of the sets? 12! That's your lowest common denominator, or LCD.
- Prime factorization. If you know what factors are, you can do prime factorization. That's finding out what numbers can make your denominators. For 3, the factors are 3 and 1. For 4, the factors are 2 and 2. Then, you multiply them together. 3 x 2 x 2 = 12. Your LCD!
  - Multiply the numbers together for small numbers. In some cases, like this one, you could just multiply the numbers together – 3 x 4 = 12. However, if your denominators are big, don't do this! You don't want to multiply 56 x 44 and have to work with 2,464 as your answer!
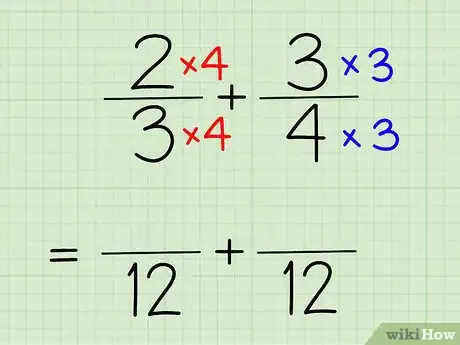
2
Multiply the denominator by the number needed to get the LCD.^{[6]
X
Research source} In other words, you want each of your denominators to be the same number – the LCD. In our example, we want our denominator to be 12. To turn 3 into 12, you need 3 x 4. To turn 4 into twelve, you need 4 x 3. The resulting like denominator will be the denominator for your final answer.
- So our 2/3 turns into 2/3 x 4 and 3/4 turns into 3/4 x 3. That means we now have 2/12 and 3/12. But we're not done yet!
  - You'll notice that the denominators, in this instance, are multiplied by each other. This works in this situation, but not all situations. Sometimes, instead of multiplying the two denominators together, you can multiply both denominators by different numbers to get one small number.
  - And then in other cases, sometimes you only have to multiply one denominator to make it equal to the denominator of the other fraction in the equation.
3
Multiple the numerator by that number, too. When you multiply the denominator by a certain number, you also have to multiply the numerator by the same number. What we did in the last step was just half of the multiplication necessary.^{[7]
X
Research source}
- We had 2/3x4 and 3/4x3 as our first step – to add the second step, it's really 2 x 4/3 x 4 and 3 x 3/4 x 3. That means our new numbers are 8/12 and 9/12. Perfect!
4
Add (or subtract) the numerators to get your answer. To add 8/12 + 9/12, all you have to do is add the numerators. Remember: you leave the denominator alone now. The number you got with the LCD is your final denominator.^{[8]
X
Research source}
- For this example, (8+9)/12 = 17/12. To turn this into a mixed fraction, simply subtract the denominator from the numerator and see what's left over. In this case, 17/12 = 1 5/12

Method 3

Method 3 of 4:

Adding and Subtracting Mixed and Improper Fractions

1
Convert your mixed fractions into improper fractions. A mixed fraction is when you have a whole number and a fraction, like in the above example (1 5/12). Meanwhile, an improper fraction is one where the numerator (the top number) is bigger than the denominator (the bottom number). That's also seen in the above step, with 17/12.^{[9]
X
Research source}
- For the example for this section, let's work with 13/12 and 17/8.
2
Find the common denominator. Remember the three ways you can find the LCD? By either writing out the multiples, using prime factorization, or by multiplying the denominators.^{[10]
X
Research source}
- Let's figure out the multiples of our example, 12 and 8. What's the smallest number these two go into? 24. 8, 16, 24 and 12, 24 – bingo!
3
Multiply your numerators and denominators to get your like fraction. Both denominators now need to be turned into 24. How do you get 12 to 24? Multiply it by 2. 8 to 24? Multiply it by three. But don't forget – you need to multiply the numerators, too!^{[11]
X
Research source}
- So 13 x 2/12 x 2 = 26/24. And 17 x 3/8 x 3 = 51/24. We're well on our way to solving the problem!
4
Add or subtract your fractions. Now that you have the same denominator, you can add these two numbers together with ease. Remember, leave the denominator alone!^{[12]
X
Research source}
- 26/24 + 51/24 = 77/24. There's your one fraction! That top number is mighty big, though....
5
Convert your answer back into a mixed fraction. Having such a large number on top is a little weird – you can't quite tell the size of your fraction. All you have to do is put the denominator into the numerator until in can't be repeated again and then see what you have leftover.^{[13]
X
Research source}
- For this example, 24 goes into 77 three times. That is, 24 x 3 = 72. But there's 5 leftover! So what's your final answer? 3 5/24. That's it!

Method 4

Method 4 of 4:

Adding and Subtracting Fractions without looking for the LCD

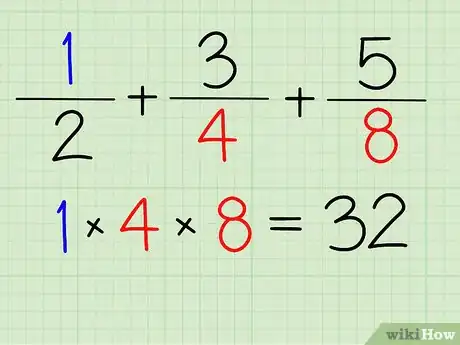
1
List the fractions.
- e.g. ½ + ¾ + ⅝
2
Solve for the numerators first.^{[14]
X
Research source}
- Multiply ¹ to the denominator/s of the other fractions.
- Multiply 1 to 4 and 8. [32]
3
Do as to other fraction.
- Multiply 3 with 2 and 8. [48]
- Lastly, multiply 5 with 4 and 2. [40]
4
Add all the product.
- 32+48+40=120
5
Now you have the numerator.
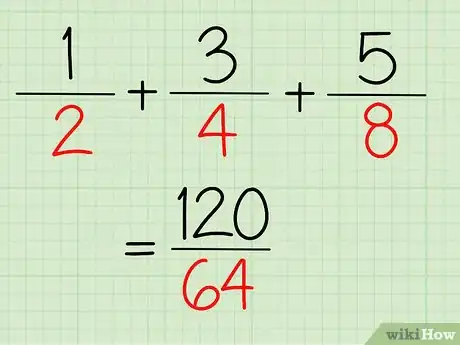
6
Solve for the denominator.^{[15]
X
Research source}
7
Multiply all the denominator.
- 2×4×8=64
8
You have the answer.
- 120/64 = 1 56/64 = 1 ⅞

Calculator, Practice Problems, and Answers

Sample Adding and Subtracting Fractions Calculator

Sample Adding and Subtracting Fractions Practice Problems

Sample Adding and Subtracting Fractions Practice Answers

Community Q&A

Question

Why do I have to multiply and find a common denominator?

Donagan

Top Answerer

Think of adding or subtracting fractions as a bit like adding or subtracting apples and oranges. You can't add 1 apple to 2 oranges unless you call each of them "pieces of fruit." Then if you added them, you'd have 3 pieces of fruit. Similarly you can't add 1/4 to 1/3 unless you re-name each fraction such that they have the same denominator: 1/4 becomes 3/12, and 1/3 becomes 4/12. Adding them together simply involves adding the numerators to get 7/12. Subtracting is similar, except you subtract one numerator from the other.
Question

I'm trying to work this out. I know the answer because I used my calculator, but whats the formula for 7/9 - 1/6 + 8/12?

Donagan

Top Answerer

First reduce fractions. The only one that will reduce is 8/12, reducing to 2/3. Next find the lowest (or least) common denominator: the LCD is 9 x 6 = 54. Then 7/9 = 42/54, 1/6 = 9/54, and 2/3 = 36/54. So 42/54 - 9/54 + 36/54 = 69/54 = 1 15/54.
Question

What about 5 1/2 - 2 2/3?

Donagan

Top Answerer

Convert 5½ to 11/2 and then to 33/6. Convert 2 2/3 to 8/3 and then to 16/6. 33/6 - 16/6 = 17/6 = 2 5/6.