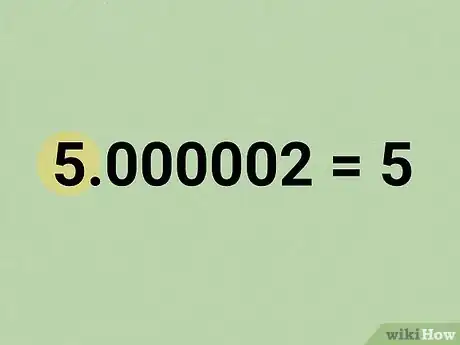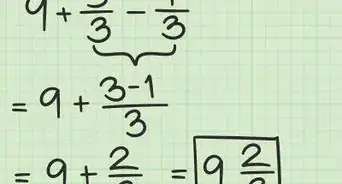This article was co-authored by wikiHow Staff. Our trained team of editors and researchers validate articles for accuracy and comprehensiveness. wikiHow's Content Management Team carefully monitors the work from our editorial staff to ensure that each article is backed by trusted research and meets our high quality standards.
This article has been viewed 244,488 times.
Learn more...
The Fibonacci sequence is a pattern of numbers generated by summing the previous two numbers in the sequence.[1] The numbers in the sequence are frequently seen in nature and in art, represented by spirals and the golden ratio. The easiest way to calculate the sequence is by setting up a table; however, this is impractical if you are looking for, for example, the 100th term in the sequence, in which case Binet’s formula can be used.
Steps
Using a Table
-
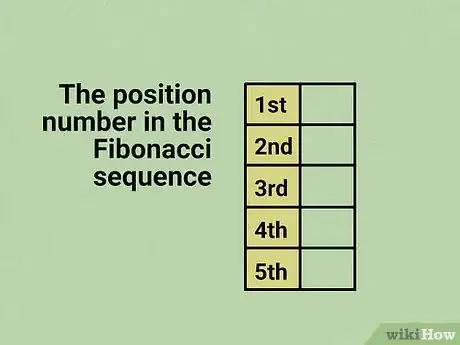
1Set up a table with two columns. The number of rows will depend on how many numbers in the Fibonacci sequence you want to calculate.[2]
- For example, if you want to find the fifth number in the sequence, your table will have five rows.
- When using the table method, you cannot find a random number farther down in the sequence without calculating all the number before it. For example, if you want to find the 100th number in the sequence, you have to calculate the 1st through 99th numbers first. This is why the table method only works well for numbers early in the sequence.
-
2Enter the sequence of terms in the left column. This means just entering a sequence of sequential ordinal numbers, beginning with "1st."
- The term refers to the position number in the Fibonacci sequence.
- For example, if you want to figure out the fifth number in the sequence, you will write 1st, 2nd, 3rd, 4th, 5th down the left column. This will show you what the first through fifth terms in the sequence are.
Advertisement -
3Enter 1 in the first row of the right-hand column. This is the starting point for the Fibonacci Sequence. In other words, the first term in the sequence is 1.
- The correct Fibonacci sequence always starts on 1. If you begin with a different number, you are not finding the proper pattern of the Fibonacci sequence.
-
4Add the first term (1) and 0. This will give you the second number in the sequence.
- Remember, to find any given number in the Fibonacci sequence, you simply add the two previous numbers in the sequence.
- To create the sequence, you should think of 0 coming before 1 (the first term), so 1 + 0 = 1.
-
5Add the first term (1) and the second term (1). This will give you the third number in the sequence.[3]
- 1 + 1 = 2. The third term is 2.
-
6Add the second term (1) and the third term (2) to get the fourth number in the sequence.
- 1 + 2 = 3. The fourth term is 3.
-
7Add the third term (2) and the fourth term (3). This will give you the fifth number in the sequence.[4]
- 2 + 3 = 5. The fifth term is 5.
-
8
Using Binet's Formula and the Golden Ratio
-
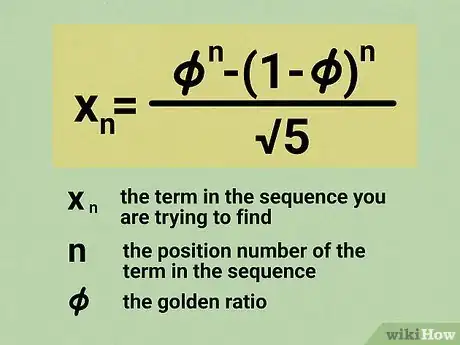
1Set up the formula =. In the formula, = the term in the sequence you are trying to find, = the position number of the term in the sequence, and = the golden ratio.[7]
- This is a closed formula, so you will be able to calculate a specific term in the sequence without calculating all the previous ones.
- This formula is a simplified formula derived from Binet’s Fibonacci number formula.[8]
- The formula utilizes the golden ratio (), because the ratio of any two successive numbers in the Fibonacci sequence are very similar to the golden ratio.[9]
-
2Plug the number for into the formula. The represents whatever term you are looking for in the sequence.
- For example, if you are looking for the fifth number in the sequence, plug in 5. Your formula will now look like this: =.
-
3Substitute the golden ratio into the formula. You can use 1.618034 as an approximation of the golden ratio.[10]
- For example, if you are looking for the fifth number in the sequence, the formula will now look like this: =.
-
4Complete the calculations in parentheses. Remember to use the order of operations by completing the calculation in parentheses first: .
- In the example, the equation becomes =.
-
5Calculate the exponents. Multiply the two parenthetical numbers in the numerator by the appropriate exponent.
- In the example, ; . So the equation becomes .
-
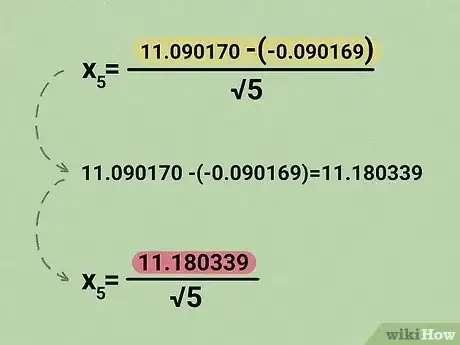
6Complete the subtraction. Before you divide, you need to subtract the two numbers in the numerator.
- In the example, , so the equation becomes =.
-
7Divide by the square root of 5. The square root of 5, rounded, is 2.236067.[11]
- In the example problem, .
-
8Round to the nearest whole number. Your answer will be a decimal, but it will be very close to a whole number. This whole number represents the number in the Fibonacci sequence.
- If you used the complete golden ratio and did no rounding, you would get a whole number. It’s more practical to round, however, which will result in a decimal.[12]
- In the example, after using a calculator to complete all the calculations, your answer will be approximately 5.000002. Rounding to the nearest whole number, your answer, representing the fifth number in the Fibonacci sequence, is 5.
Community Q&A
-
QuestionIs "Fibonacci" an English word?
 DanoyachtcaptTop AnswererNo, it is the name of mathematician Leonardo of Pisa.
DanoyachtcaptTop AnswererNo, it is the name of mathematician Leonardo of Pisa. -
QuestionHow do I deduce Binet's fibonacci number formula?
 OrangejewsCommunity AnswerOne way is to interpret the recursion as a matrix multiplication. Take a vector of two consecutive terms like (13, 8), multiply by a transition matrix M = (1,1; 1,0) to get the next such vector (21,13). That gives a formula involving M^n, but if you diagonalize M, computing M^n is easy and that formula pops right out.
OrangejewsCommunity AnswerOne way is to interpret the recursion as a matrix multiplication. Take a vector of two consecutive terms like (13, 8), multiply by a transition matrix M = (1,1; 1,0) to get the next such vector (21,13). That gives a formula involving M^n, but if you diagonalize M, computing M^n is easy and that formula pops right out. -
QuestionWho discovered this sequence?
 WOOHPCommunity AnswerLeonardo Bonacci
WOOHPCommunity AnswerLeonardo Bonacci
References
- ↑ https://mathworld.wolfram.com/FibonacciNumber.html
- ↑ https://www.mathsisfun.com/numbers/fibonacci-sequence.html
- ↑ https://www.cuemath.com/algebra/fibonacci-numbers/
- ↑ https://www.rapidtables.com/math/number/fibonacci.html
- ↑ http://mathworld.wolfram.com/FibonacciNumber.html
- ↑ https://www.cuemath.com/algebra/fibonacci-numbers/
- ↑ https://www.mathsisfun.com/numbers/fibonacci-sequence.html
- ↑ http://mathworld.wolfram.com/FibonacciNumber.html
- ↑ https://www.mathsisfun.com/numbers/fibonacci-sequence.html
- ↑ https://www.mathsisfun.com/numbers/fibonacci-sequence.html
- ↑ https://math.libretexts.org/Bookshelves/Applied_Mathematics/Book%3A_College_Mathematics_for_Everyday_Life_(Inigo_et_al)/10%3A_Geometric_Symmetry_and_the_Golden_Ratio/10.04%3A_Fibonacci_Numbers_and_the_Golden_Ratio
- ↑ https://www.mathsisfun.com/numbers/fibonacci-sequence.html
About This Article
To calculate the Fibonacci sequence up to the 5th term, start by setting up a table with 2 columns and writing in 1st, 2nd, 3rd, 4th, and 5th in the left column. Next, enter 1 in the first row of the right-hand column, then add 1 and 0 to get 1. Write 1 in the column next to “2nd,” then add the 1st and 2nd term to get 2, which is the 3rd number in the sequence. Continue this pattern of adding the 2 previous numbers in the sequence to get 3 for the 4th term and 5 for the 5th term. To learn more, including how to calculate the Fibonacci sequence using Binet’s formula and the golden ratio, scroll down.