Unlike the other orders of reaction, a zero-order reaction has a rate that is independent of the concentration of the reactant(s). As such, increasing or decreasing the concentration of the reacting species will not speed up or slow down the reaction rate. Zero-order reactions are typically found when a material that is required for the reaction to proceed, such as a surface or a catalyst, is saturated by the reactants.
The rate law for a zero-order reaction is rate = k, where k is the rate constant. In the case of a zero-order reaction, the rate constant k will have units of concentration/time, such as M/s.
Plot of Concentration Versus Time for a Zero-Order Reaction
Recall that the rate of a chemical reaction is defined in terms of the change in concentration of a reactant per change in time. This can be expressed as follows:
By rearranging this equation and using a bit of calculus (see the next concept: The Integrated Rate Law), we get the equation:
This is the integrated rate law for a zero-order reaction. Note that this equation has the form
Half-Life of a Zero-Order Reaction
The half-life of a reaction describes the time needed for half of the reactant(s) to be depleted, which is the same as the half-life involved in nuclear decay, a first-order reaction. For a zero-order reaction, the half-life is given by:
[A]0 represents the initial concentration and k is the zero-order rate constant.
Example of a Zero-Order Reaction
The Haber process is a well-known process used to manufacture ammonia from hydrogen and nitrogen gas. The reverse of this is known, simply, as the reverse Haber process, and it is given by:
The reverse Haber process is an example of a zero-order reaction because its rate is independent of the concentration of ammonia. As always, it should be noted that the order of this reaction, like the order for all chemical reactions, cannot be deduced from the chemical equation, but must be determined experimentally.
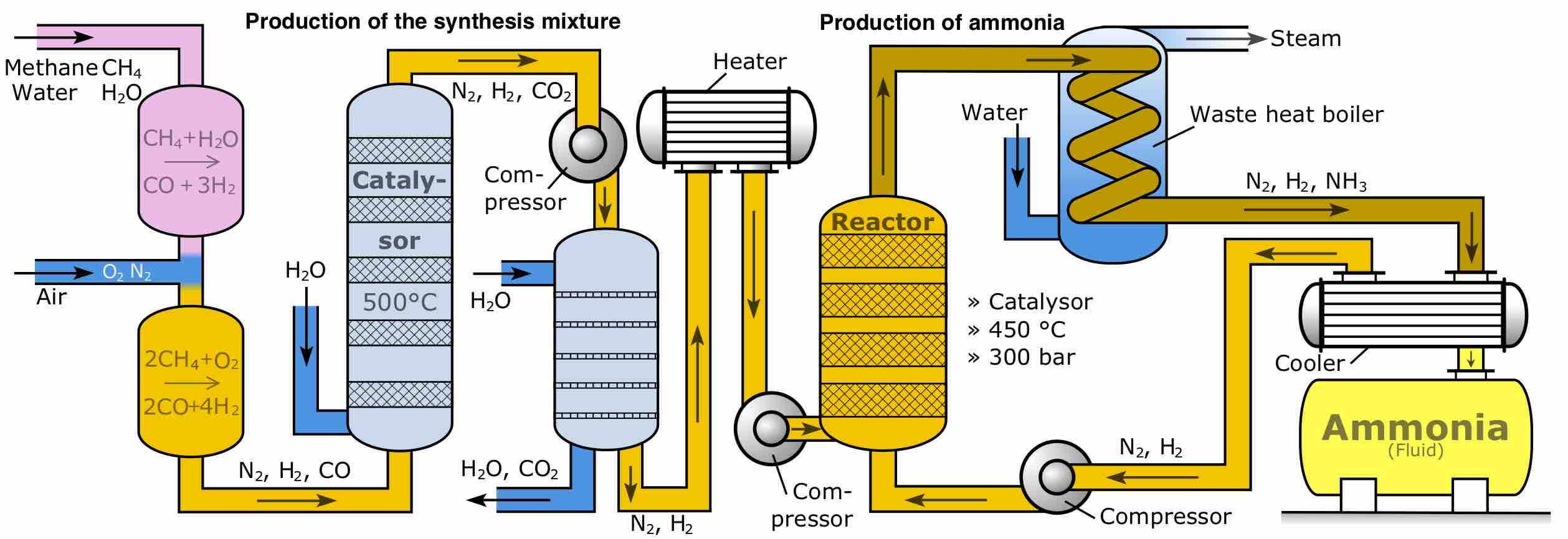
The Haber process
The Haber process produces ammonia from hydrogen and nitrogen gas. The reverse of this process (the decomposition of ammonia to form nitrogen and hydrogen) is a zero-order reaction.