Step By Step Example of a Confidence Interval for a Mean—Standard Deviation Known
Suppose scores on exams in statistics are normally distributed with an unknown population mean, and a population standard deviation of 3 points. A random sample of 36 scores is taken and gives a sample mean (sample mean score) of 68. To find a 90% confidence interval for the true (population) mean of statistics exam scores, we have the following guidelines:
- Plan: State what we need to know.
- Model: Think about the assumptions and check the conditions.
- State the parameters and the sampling model.
- Mechanics:
$\text{CL} = 0.90$ , so$\alpha = 1-\text{CL} = 1-0.90 = 0.10$ ;$\alpha_{0.05}$ is$1-0.05 = 0.95$ ; So$z_{0.05} = 1.645$ - Conclusion: Interpret your result in the proper context, and relate it to the original question.
1. In our example, we are asked to find a 90% confidence interval for the mean exam score,
We have a sample of 68 students.
2. We know the population standard deviation is 3. We have the following conditions:
- Randomization Condition: The sample is a random sample.
- Independence Assumption: It is reasonable to think that the exam scores of 36 randomly selected students are independent.
- 10% Condition: We assume the statistic student population is over 360 students, so 36 students is less than 10% of the population.
- Sample Size Condition: Since the distribution of the stress levels is normal, our sample of 36 students is large enough.
3. The conditions are satisfied and
4. below shows the steps for calculating the confidence interval.
The 90% confidence interval for the mean score is
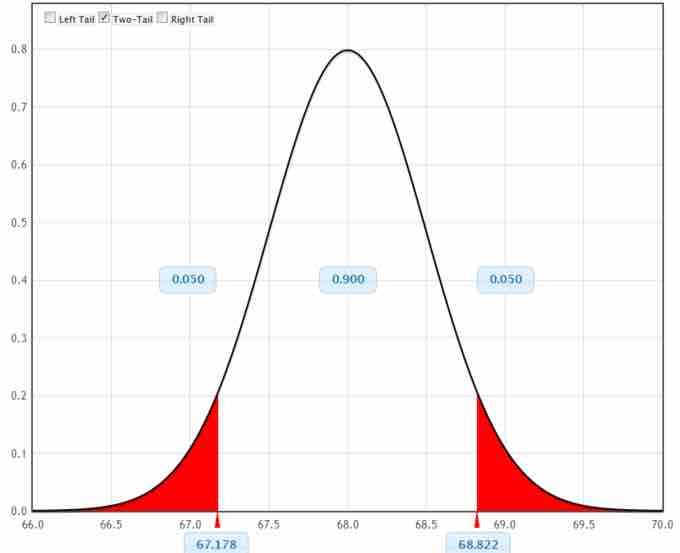
Graphical Representation
This figure is a graphical representation of the confidence interval we calculated in this example.
5. In conclusion, we are 90% confident that the interval from 67.1775 to 68.8225 contains the true mean score of all the statistics exams. 90% of all confidence intervals constructed in this way contain the true mean statistics exam score.