Volume Expansion

The volumetric thermal expansion coefficient is the most basic thermal expansion coefficient. illustrates that, in general, substances expand or contract when their temperature changes, with expansion or contraction occurring in all directions. Such substances that expand in all directions are called isotropic. For isotropic materials, the area and linear coefficients may be calculated from the volumetric coefficient (discussed below).

Volumetric Expansion

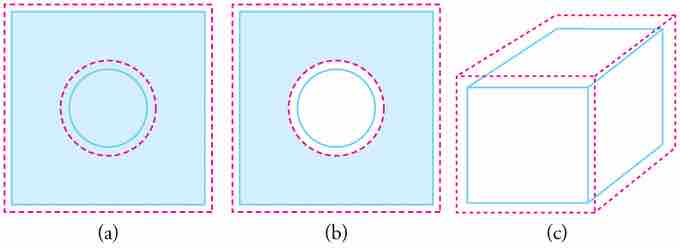
In general, objects expand in all directions as temperature increases. In these drawings, the original boundaries of the objects are shown with solid lines, and the expanded boundaries with dashed lines. (a) Area increases because both length and width increase. The area of a circular plug also increases. (b) If the plug is removed, the hole it leaves becomes larger with increasing temperature, just as if the expanding plug were still in place. (c) Volume also increases, because all three dimensions increase.

Mathematical definitions of these coefficients are defined below for solids, liquids, and gasses:

$\alpha_V= \frac{1}{V} (\frac{\partial V}{\partial T})_p$.

The subscript p indicates that the pressure is held constant during the expansion. In the case of a gas, the fact that the pressure is held constant is important, as the volume of a gas will vary appreciably with pressure as well as with temperature.

For a solid, we can ignore the effects of pressure on the material, thus the volumetric thermal expansion coefficient can be written:

$\alpha_V = \frac{1}{V} \frac{dV}{dT}$,

where V is the volume of the material, and is dV/dT the rate of change of that volume with temperature. This means that the volume of a material changes by some fixed fractional amount. For example, a steel block with a volume of 1 cubic meter might expand to 1.002 cubic meters when the temperature is raised by 50 °C. This is an expansion of 0.2%. The volumetric expansion coefficient would be 0.2% for 50 °C, or 0.004% per degree C.

Relationship to Linear Thermal Expansion Coefficient

For isotropic material, and for small expansions, the linear thermal expansion coefficient is one third the volumetric coefficient. To derive the relationship, let's take a cube of steel that has sides of length L. The original volume will be V = L³,and the new volume, after a temperature increase, will be:

$\begin{aligned} V+ \Delta V &= (L + \Delta L)^3 \\ &= L^3 + 3L^2\Delta L + 3L(\Delta L )^2 +(\Delta L)^3 \\ &\approx L^3 + 3L^2\Delta L \\ &= V + 3 V \frac {\Delta L}{L} \end{aligned}$.

The approximation holds for a sufficiently small $\Delta L$ compared to L. Since:

$\frac{\Delta V}{V} = 3\frac{\Delta L}{L}$

(and from the definitions of the thermal coefficients), we arrive at:

$\alpha_V =3 \alpha_L$.