The Definite Integral

Integration is an important concept in mathematics and—together with its inverse, differentiation—is one of the two main operations in calculus. Given a function $f$ of a real variable x and an interval $[a, b]$ of the real line, the definite integral $\int_{a}^{b}f(x)dx$ is defined informally to be the area of the region in the $xy$-plane bound by the graph of $f$, the $x$-axis, and the vertical lines $x = a$ and $x=b$, such that the area above the $x$-axis adds to the total, and that the area below the $x$-axis subtracts from the total. Integrals such as these are termed definite integrals.

Definite Integral

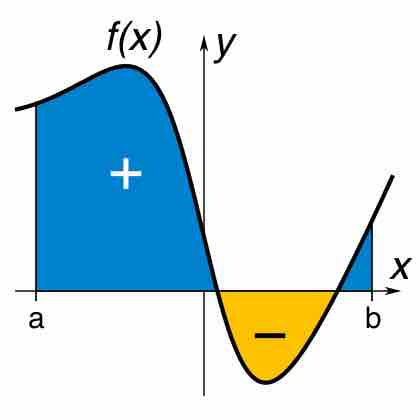
A definite integral of a function can be represented as the signed area of the region bounded by its graph.

The principles of integration were formulated independently by Isaac Newton and Gottfried Leibniz in the late 17th century. Through the fundamental theorem of calculus, which they independently developed, integration is connected with differentiation: if $f$ is a continuous real-valued function defined on a closed interval $[a, b]$, then, once an antiderivative $F$ of $f$ is known, the definite integral of $f$ over that interval is given by

$\displaystyle{\int_{a}^{b}f(x)dx = F(b) - F(a)}$

Definite integrals appear in many practical situations. If a swimming pool is rectangular with a flat bottom, then from its length, width, and depth we can easily determine the volume of water it can contain (to fill it), the area of its surface (to cover it), and the length of its edge (to rope it). But if it is oval with a rounded bottom, all of these quantities call for integrals. Practical approximations may suffice for such trivial examples, but precision engineering (of any discipline) requires exact and rigorous values for these elements.

For example, consider the curve $y = f(x)$ between 0 and x = 1 with $f(x) = \sqrt{x}.$

We ask, "What is the area under the function $f$, over the interval from 0 to 1? " and call this (yet unknown) area the integral of $f$. The notation for this integral will be:

$\int_{0}^{1} \sqrt{x} dx$

As a first approximation, look at the unit square given by the sides $x = 0$ to $x = 1$, $y = f(0) = 0$, and $y = f(1) = 1$. Its area is exactly $1$. As it is, the true value of the integral must be somewhat less. Decreasing the width of the approximation rectangles should yield a better result, so we will cross the interval in five steps, using the approximation points $0$, $\frac{1}{5}$, $\frac{2}{5}$, and so on, up to $1$. Fit a box for each step using the right end height of each curve piece, thus obtaining $\sqrt{\frac{1}{5}}$, $\sqrt{\frac{2}{5}}$, and so on, up to$\sqrt{1} = 1$. Summing the areas of these rectangles, we get a better approximation for the sought integral, namely:

$\displaystyle{\sqrt{\frac{1}{5}} \left ( \frac{1}{5} - 0 \right ) + \sqrt{\frac{2}{5}} \left ( \frac{2}{5} - \frac{1}{5} \right ) + \cdots + \sqrt{\frac{5}{5}} \left ( \frac{5}{5} - \frac{4}{5} \right ) \approx 0.7497}$

Notice that we are taking a finite sum of many function values of $f$, multiplied with the differences of two subsequent approximation points. We can easily see that the approximation is still too large. Using more steps produces a closer approximation, but will never be exact: replacing the $5$ subintervals by twelve as depicted, we will get an approximate value for the area of $0.6203$, which is too small. The key idea is the transition from adding a finite number of differences of approximation points multiplied by their respective function values to using an infinite number of fine, or infinitesimal, steps.

As for the actual calculation of integrals, the fundamental theorem of calculus, due to Newton and Leibniz, is the fundamental link between the operations of differentiating and integrating. Applied to the square root curve, $f(x) = x^{1/2}$, the theorem says to look at the antiderivative:

$\displaystyle{F(x) = \frac{2}{3} \cdot x^\frac{3}{2}}$

and simply take $F(1) − F(0)$, where $0$ and $1$ are the boundaries of the interval $[0,1]$. So the exact value of the area under the curve is computed formally as:

$\displaystyle{\int_{0}^{1}\sqrt{x}dx = \int_{0}^{1}x^{1/2}dx = F(1) - F(0) = \frac{2}{3}}$