The Three Dimensional Coordinate System
A three dimensional space has three geometric parameters:
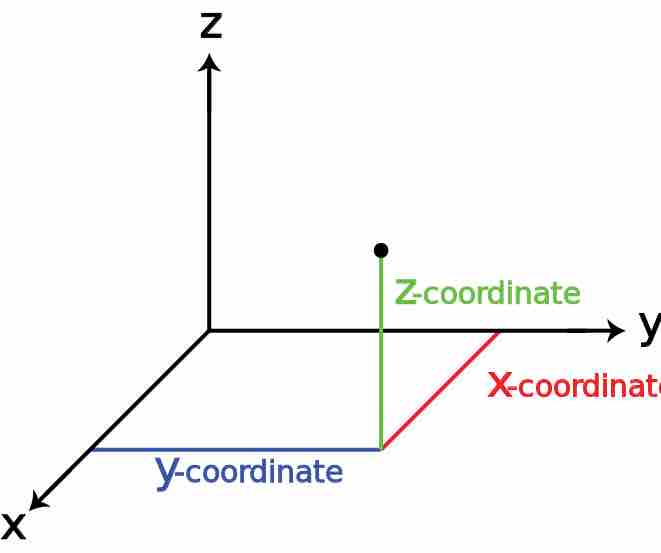
Three-Dimensional Space
This is a three dimensional space represented by a Cartesian coordinate system.
Cartesian Geometry
Also known as analytical geometry, this system is used to describe every point in three dimensional space in three parameters, each perpendicular to the other two at the origin. Each parameter is labeled relative to its axis with a quantitative representation of its distance from its plane of reference, which is determined by the other two parameter axes.
Other Coordinate Systems
Cylindrical Coordinates ($\rho, \varphi, z$ )
The cylindrical system uses two linear parameters and one radial parameter:
-
$\rho$ : the radial distance from the point to$z$ -
$\varphi$ : the angle between the reference direction and the point -
$z$ : the distance from the reference plane to the point
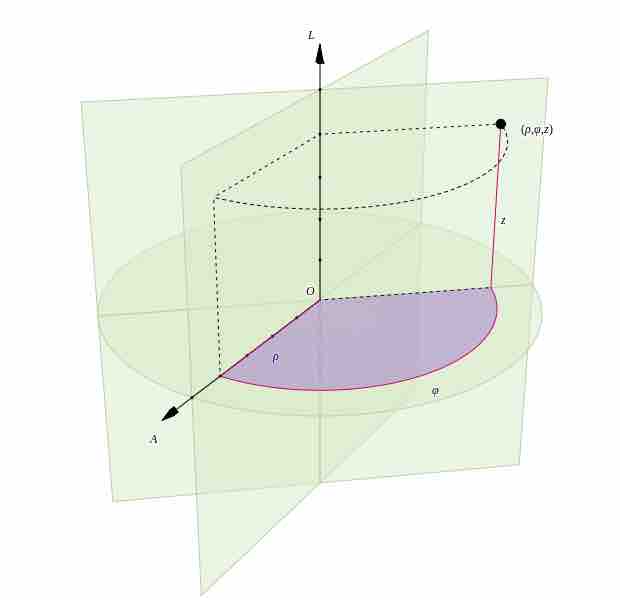
Cylindrical Coordinate System
The cylindrical coordinate system is like a mix between the spherical and Cartesian system, incorporating linear and radial parameters.
Spherical Coordinates ($r$ , $\theta$ , $\varphi$ )
The spherical system is used commonly in mathematics and physics:
-
$r$ : the radial distance from the origin to the point -
$\theta$ : the angle between the zenith direction and directional vector of$r$ -
$\varphi$ : the angle from the reference direction to the orthogonal plane projected by the directional vector of$r$
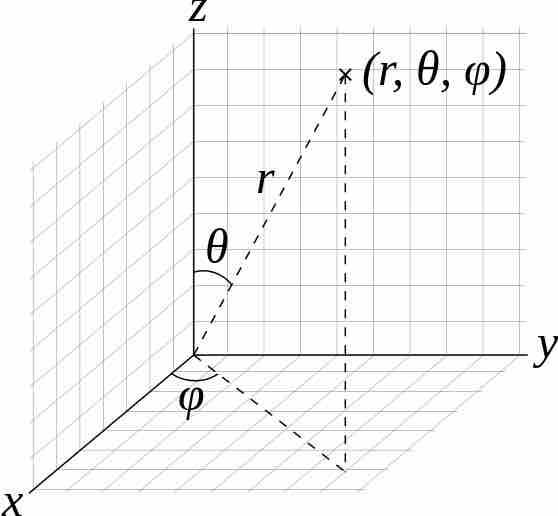
Spherical Coordinate System
The spherical system is used commonly in mathematics and physics and has variables of
Cartesian to Spherical
Often, you will need to be able to convert from spherical to Cartesian, or the other way around. The following equations will allow you to do just that: