For a sequence
is non-decreasing. Because the partial sum
Example 1
The series
and because:
Example 2
Would the series
converge? It is possible to "visualize" its convergence on the real number line? We can imagine a line of length
Therefore:
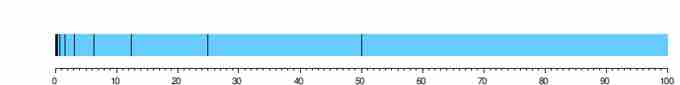
Geometric Sum
Visualization of the geometric sum in Example 2. The length of the line (
For these specific examples, there are easy ways to check the convergence. However, it could be the case that there are no easy ways to check the convergence. For these general cases, we can experiment with several well-known convergence tests (such as ratio test, integral test, etc.). We will learn some of these tests in the following atoms.