Summing an Infinite Series

A series is the sum of the terms of a sequence. Finite sequences and series have defined first and last terms, whereas infinite sequences and series continue indefinitely. Unlike finite summations, infinite series need tools from mathematical analysis, and specifically the notion of limits, to be fully understood and manipulated. In addition to their ubiquity in mathematics, infinite series are also widely used in other quantitative disciplines such as physics, computer science, and finance.

For any infinite sequence of real or complex numbers, the associated series is defined as the ordered formal sum

$\displaystyle{\sum_{n=0}^{\infty}a_n = a_0 + a_1 + a_2 + \cdots}$

The sequence of partial sums ${S_k}$ associated to a series $\sum_{n=0}^\infty a_n$ is defined for each k as the sum of the sequence ${a_n}$ from $a_0$ to $a_k$:

$\displaystyle{S_k = \sum_{n=0}^{k}a_n = a_0 + a_1 + \cdots + a_k}$

Infinite sequences and series can either converge or diverge. A series is said to converge when the sequence of partial sums has a finite limit. By definition the series $\sum_{n=0}^\infty a_n$ converges to a limit $L$ if and only if the associated sequence of partial sums  converges to $L$. This definition is usually written as: 

$\displaystyle{L = \sum_{n=0}^{\infty}a_n \Leftrightarrow L = \lim_{k \rightarrow \infty} S_k}$

If the limit of is infinite or does not exist, the series is said to diverge.

Infinite Series

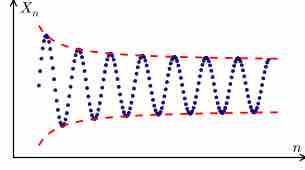
An infinite sequence of real numbers shown in blue dots. This sequence is neither increasing, nor decreasing, nor convergent, nor Cauchy. It is, however, bounded.

An easy way that an infinite series can converge is if all the $a_{n}$ are zero for sufficiently large $n$s. Such a series can be identified with a finite sum, so it is only infinite in a trivial sense. Working out the properties of the series that converge even if infinitely many terms are non-zero is, therefore, the essence of the study of series. In the following atoms, we will study how to tell whether a series converges or not and how to compute the sum of a series when such a value exists.