Change of Variables

The limits of integration are often not easily interchangeable (without normality or with complex formulae to integrate). One makes a change of variables to rewrite the integral in a more "comfortable" region, which can be described in simpler formulae. To do so, the function must be adapted to the new coordinates.

For example, for the function $f(x, y) = (x-1)^2 +\sqrt y$, if one adopts this substitution $x' = x-1, \ y'= y$, therefore $x = x' + 1, \ y=y'$, one obtains the new function:

$f_2(x,y) = (x')^2 +\sqrt y$

which is simpler than the original form. When changing integration variables, however, make sure that the integral domain also changes accordingly. In the example, if integration is performed over $x$ in $[0,1]$] and y in $[0,3]$, the new variables $x'$ and $y'$ vary over $[-1,0]$ and $[0,3]$, respectively. There exist three main "kinds" of changes of variable (one in $R^2$, two in $R^3$); however, more general substitutions can be made using the same principle.

1. Polar coordinates

The function to be integrated transforms as:

$f(x,y) \rightarrow f(\rho \cos \phi,\rho \sin \phi )$

and the integral accordingly changes as:

$\displaystyle {\iint_D f(x,y) \ dx\, dy = \iint_T f(\rho \cos \phi, \rho \sin \phi) \rho \, d \rho\, d \phi}$

2. Cylindrical coordinates

The function to be integrated transforms as:

$f(x,y,z) \rightarrow f(\rho \cos \phi, \rho \sin \phi, z)$

and the integral accordingly changes as:

$\displaystyle {\iiint_D f(x,y,z) \, dx\, dy\, dz = \iiint_T f(\rho \cos \phi, \rho \sin \phi, z) \rho \, d\rho\, d\phi\, dz}$

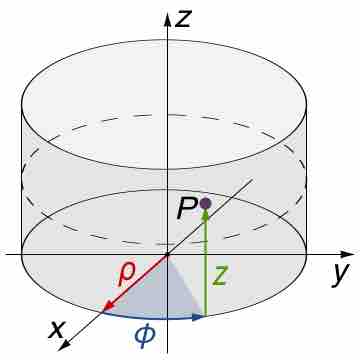
Cylindrical Coordinates

Changing to cylindrical coordinates may be useful depending on the setup of problem.

3. Spherical coordinates

The function to be integrated transforms as:

 $f(x,y,z) \longrightarrow f(\rho \cos \theta \sin \phi, \rho \sin \theta \sin \phi, \rho \cos \phi)$

and the integral accordingly changes as:

$\displaystyle {\iiint_D f(x,y,z) \, dx\, dy\, dz \\ = \iiint_T f(\rho \sin \phi \cos \theta, \rho \sin \phi \sin \theta, \rho \cos \phi) \rho^2 \sin \phi \, d\rho\, d\theta\, d\phi}$