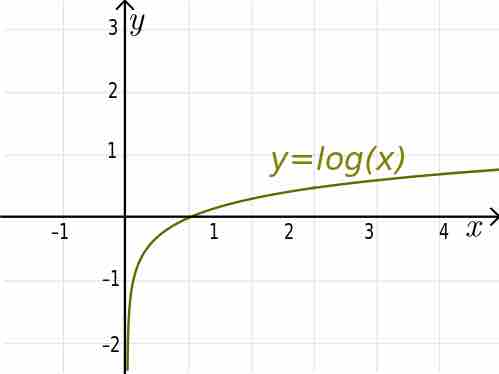
Below is the graph of the $y=logx$. Recall that this is the common log and has a base of $10$.

$y=\log x$

The graph of the base-$10$ logarithm

The logarithmic graph begins with a steep climb after $x=0$, but stretches more and more horizontally, its slope ever-decreasing as $x$ increases. 

Properties of the Graphs of Logarithmic Functions 

Special Points

The graph crosses the $x$-axis at $1$. That is, the graph has an $x$-intercept of $1$, and as such, the point $(1,0)$ is on the graph. In fact, the point $(1,0)$ will always be on the graph of a function of the form $y=log{_b}x$ where $b>0$. This is because for $x=1$, the equation of the graph becomes $y=log{_b}1$. 

Thus, we are looking for an exponent $y$ such that $b^y=1$. As $b>0$, the exponent we seek is $1$ irrespective of the value of $b$. This means the point $(x,y)=(1,0)$ will always be on a logarithmic function of this type.

Asymptotes

The $y$-axis is a vertical asymptote of the graph. This means that the curve gets closer and closer to the $y$-axis but does not cross it. 

Let us consider what happens as the value of $x$ approaches zero from the right for the equation whose graph appears above. Namely, $y=log{_b}x$. We can do this by choosing values for $x$, plugging them into the equation and generating values for $y$. 

Let us assume that $b$ is a positive number greater than $1$, and let us investigate values of $x$ between $0$ and $1$. Under these conditions, if we let $x=\frac{1}{b}$, the equation becomes $y=log\frac{1}{b}$. 

Thus, we are looking for an exponent such that $b$ raised to that exponent yields $\frac{1}{b}$. The exponent we seek is $-1$ and the  point $(\frac{1}{b},-1)$ is on the graph. Similarly, we can obtain the following points that are also on the graph:

$(\frac{1}{b^2},-2),(\frac{1}{b^3},-3),(\frac{1}{b^4},-4)$ and so on 

If we take values of $x$ that are even closer to $0$, we can arrive at the following points: $(\frac{1}{b^{10}},-10),(\frac{1}{b^{100}},-100)$ and $(\frac{1}{b^{1000}},-1000)$ 

As can be seen the closer the value of $x$ gets to $0$, the more and more negative the graph becomes. That is, as $x$ approaches zero the graph approaches negative infinity. This means that the $y$-axis is a vertical asymptote of the function.

Domain and Range

The domain of the function is all positive numbers. That means that the $x$-value of the function will always be positive. Let us begin by considering why the $x$-value of the curve is never $0$. 

If the $x$-value were zero, the function would read $y=log{_b}0$. 

Here we are looking for an exponent such that $b$ raised to that exponent is $0$. Since $b$ is a positive number, there is no exponent that we can raise $b$ to so as to obtain $0$. In fact, since $b$ is positive, raising it to a power will always yield a positive number.

The range of the function is all real numbers. That is, the graph can take on any real number.

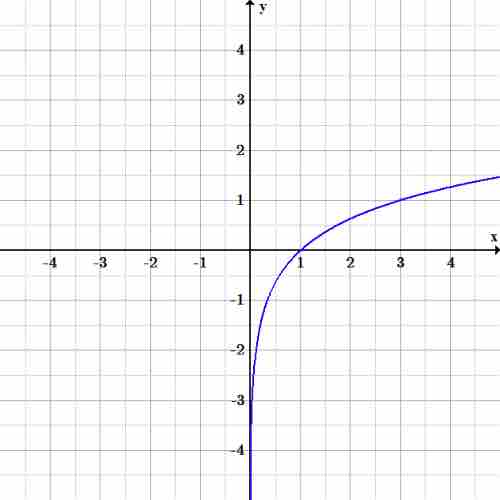
Comparing $y=log{_x}$ and $y=\sqrt{x}$

At first glance, the graph of the logarithmic function can easily be mistaken for that of the square root function.

Graph of $y=\sqrt{x}$

The graph of the square root function resembles the graph of the logarithmic function, but does not have a vertical asymptote.

Both the square root and logarithmic functions have a domain limited to $x$-values greater than $0$. However, the logarithmic function has a vertical asymptote descending towards $-\infty$ as $x$ approaches $0$, whereas the square root reaches a minimum $y$-value of $0$. The range of the square root function is all non-negative real numbers, whereas the range of the logarithmic function is all real numbers.

Graphing Logarithmic Functions

Graphing logarithmic functions can be done by locating points on the curve either manually or with a calculator. 

When graphing without a calculator, we use the fact that the inverse of a logarithmic function is an exponential function. 

When graphing with a calculator, we use the fact that the calculator can compute only common logarithms (base is $10$), natural logarithms (base is $e$) or binary logarithms (base is $2$). Of course, if we have a graphing calculator, the calculator can graph the function without the need for us to find points on the graph.

Graphing Logarithmic Functions Using Their Inverses

Logarithmic functions can be graphed by hand without the use of a calculator if we use the fact that they are inverses of exponential functions.   

Let us again consider the graph of the following function:

$y=log{_3}x$ 

This can be written in exponential form as: 

$3^y=x$

Now let us consider the inverse of this function. To do so, we interchange $x$ and $y$:

$3^x=y$ 

The exponential function $3^x=y$ is one we can easily generate points for. If we take some values for $x$ and plug them into the equation to find the corresponding values for $y$ we can obtain the following points:

$(-2,\frac{1}{9}),(-1,\frac{1}{3}),(0,1),(1,3),(2,9)$ and $(3,27)$ 

Now we must note that these points are not on the original function ($y=log{_3}x$) but rather on its inverse $3^x=y$. However, if we interchange the $x$ and $y$-coordinates of each point we will in fact obtain a list of points on the original function. 

These are:  $(\frac{1}{9},-2),(\frac{1}{3},-1),(1,0),(3,1),(9,2)$ and $(27,3)$. 

We plot and connect these points to obtain the graph of the function $y=log{_3}x$ below.

Graph of $y=log{_3}x$

The graph of the logarithmic function with base $3$ can be generated using the function's inverse. Its shape is the same as other logarithmic functions, just with a different scale.

Graphing Logarithmic Functions With Bases Between $0$ and $1$

Thus far we have graphed logarithmic functions whose bases are greater than $1$. If we instead consider logarithmic functions with a base $b$, such that $0<b<1$, we get a graph that is very similar to those we have seen already. 

In fact if $b>0$, the graph of $y=log{_b}x$ and the graph of $y=log{_\frac{1}{b}}x$ are symmetric over the $x$-axis. Thus, if we identify a point $(x,y)$ on the graph of $y=log{_b}x$, we can find the corresponding point on $y=log{_\frac{1}{b}}x$ by changing the sign of the $y$-coordinate. The corresponding point is $(x,-y)$.

Here is an example for $b=2$.

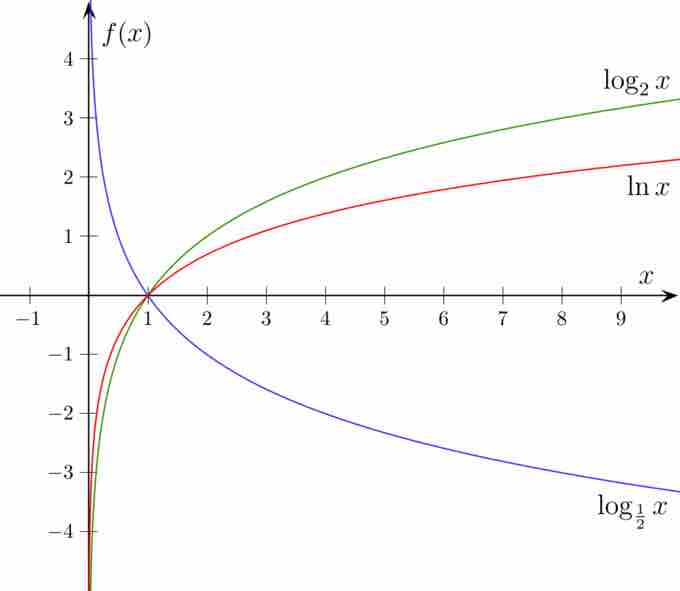
Graphs of $log{_2}x$ and $log{_\frac{1}{2}}x$ 

The graphs of $log_2 x$ and $log{_\frac{1}{2}}x$ are symmetric over the x-axis