How to Solve a 15 Puzzle

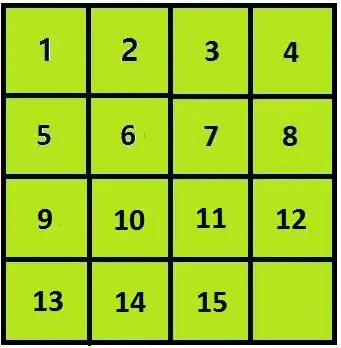
In this article, you will learn how to solve a 15 puzzle. Once you memorize the rules, your solving record can be reduced to a few minutes.

Method 1
Method 1 of 3:

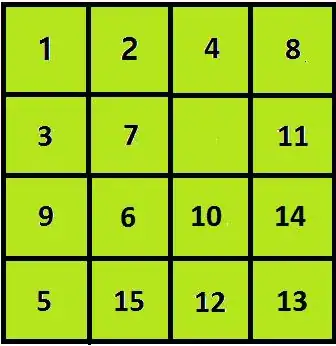
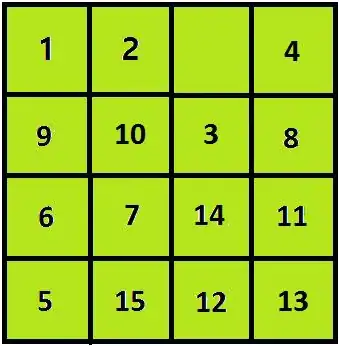
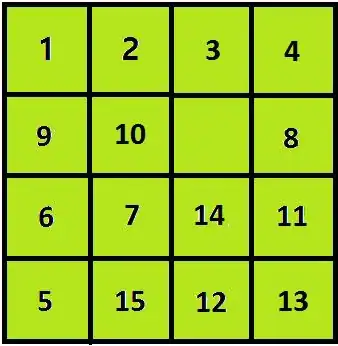
Solving the First Row

  1. Image titled Capture 21
    1
    Place 1 and 2 on their original places.
  2. Image titled 96 2
    2
    Place 4 on the right side of 2.
    Advertisement
  3. Image titled 111 15
    3
    Place 3 under 4.
  4. Image titled 666 2
    4
    Make an empty space on the right side of 4.
  5. Image titled 255 1
    5
    Push 4 to the empty space.
  6. Image titled 453 1
    6
    Push 3 upward.
    7. Advertisement
Method 2
Method 2 of 3:

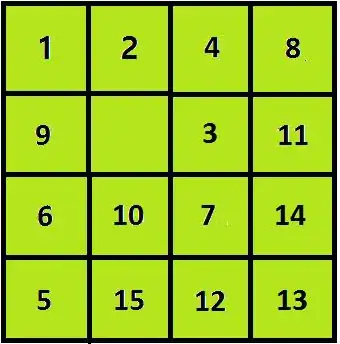
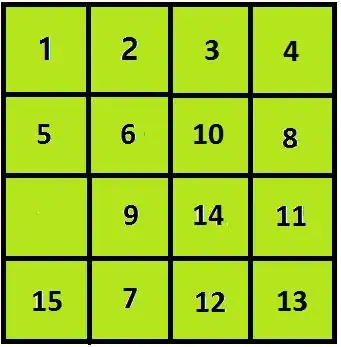
Solving the Second Row

  1. Image titled 5 147
    1
    Place 5 and 6 on their original places.
  2. Image titled 22 19
    2
    Place 8 on the right side of 6.
  3. Image titled 31 10
    3
    Place 7 under 8.
  4. Image titled 464 3
    4
    Make an empty space on the right side of 8.
  5. Image titled 33 13
    5
    Push 8 to the empty space.
  6. Image titled 55 5
    6
    Push 7 upward.
    7. Advertisement
Method 3
Method 3 of 3:

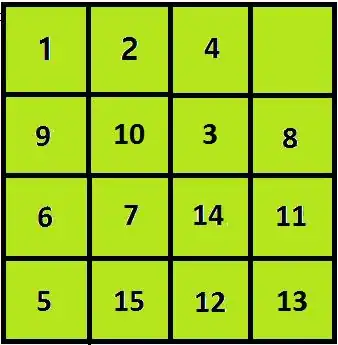
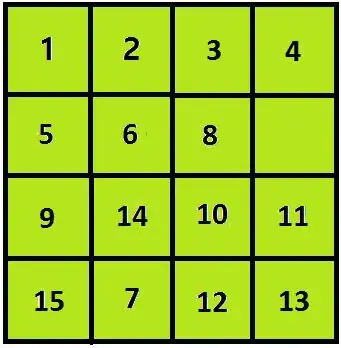
Solving the Third and Fourth Rows

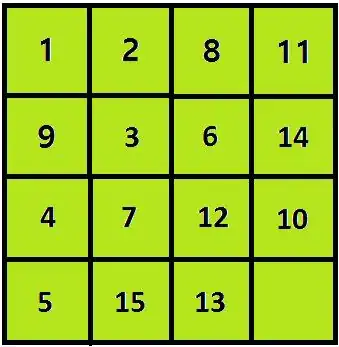
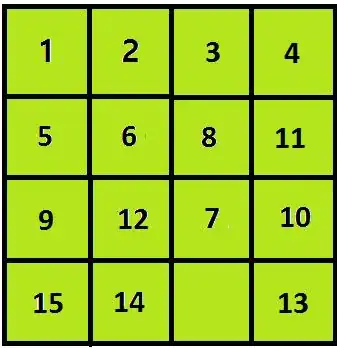
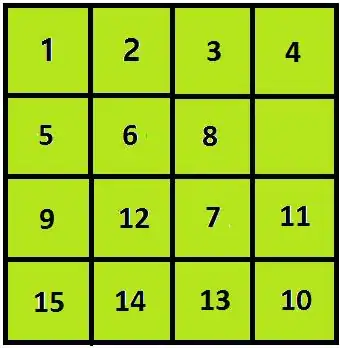
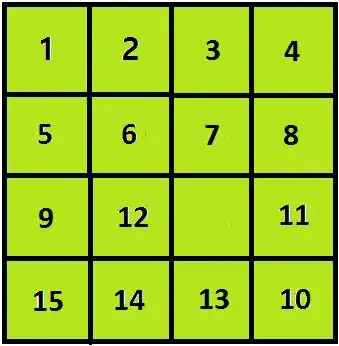
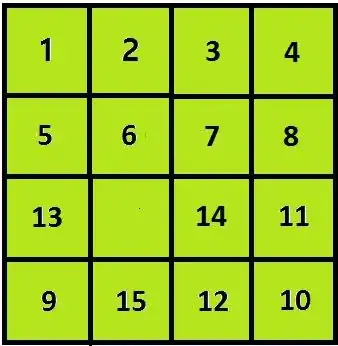
  1. Image titled 546 1
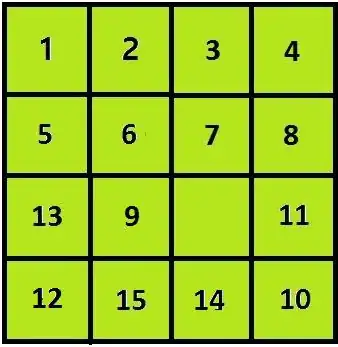
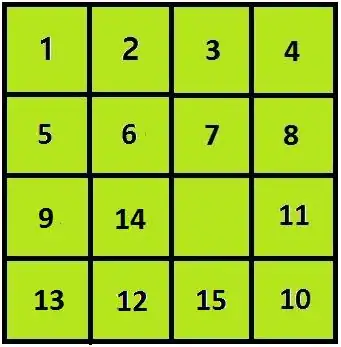
    1
    Place 13 on the original place of 9.
  2. Image titled Ds 3
    2
    Place 9 on the right side of 13.  Move 13 temporarily if you have to.
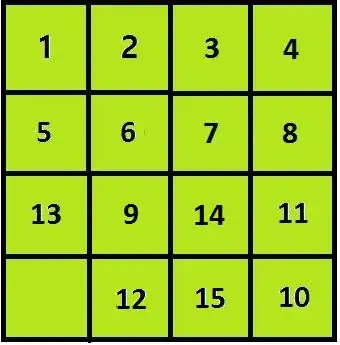
  3. Image titled 87574 3
    3
    Make an empty space under 13.
  4. Image titled Ddd 3
    4
    Push 13 downward.
  5. Image titled 4568 1
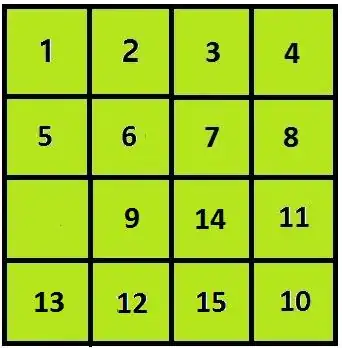
    5
    Push 9 leftward.
  6. Image titled Capture4 5
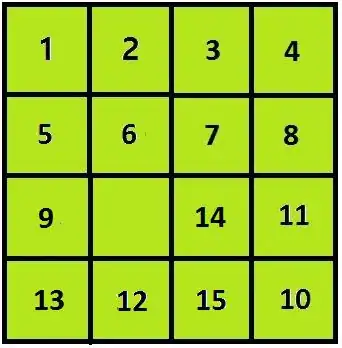
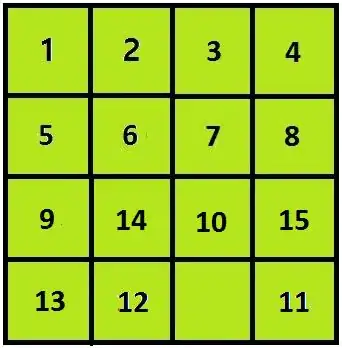
    6
    Place 14 on the original place of 10.
  7. Image titled 1111 4
    7
    Place 10 on the right side of 14.  Move 14 temporarily if you have to.
  8. Image titled Capture2 5
    8
    Make an empty space under14.
  9. Image titled Capture3 6
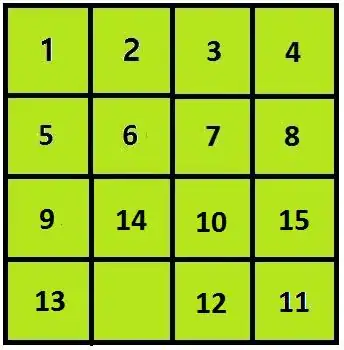
    9
    Push 14 downward.
  10. Image titled 555 4
    10
    Push 10 leftward.
  11. Image titled 875 2
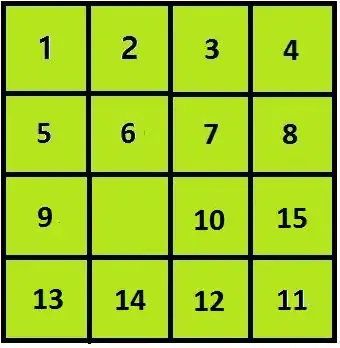
    11
    Move around the blocks 11, 12, and 15 to put them to their original places.  If they don't go to their original places, do method 3 again by mixing only the last two rows.
    12. Advertisement

Community Q&A

  • Question
    I can solve 1 through 12, but when I go on to the last row, 13 is at the end, and 14 and 15 are at the front. What am I doing wrong?
    Stephen Han
    Stephen Han
    Community Answer
    In that case, you have to do part three all over again. If you follow the steps, 13 has to be at the bottom of 9, and 14 has to be at the bottom of 10.
  • Question
    How do I change only two tiles?
    Community Answer
    Community Answer
    A single swap is known to be impossible. In this type of puzzle, exactly half of the positions are obtainable, those that require an EVEN number of swaps from the starting position.
Advertisement

You Might Also Like

Solve Hard Sudoku Puzzles
How to
Solve Hard Sudoku Puzzles
Solve Sudoku when Stuck
How to
Solve Sudoku when Stuck
Solve a Sudoku
How to
Solve a Sudoku
Solve a Magic Square3 Ways to Solve Magic Square Math Puzzles
Do Sudoku Fast
How to
Do Sudoku Fast
Play Sudoku for Kids
How to
Play Sudoku for Kids
How to
Create a Sudoku
Solve 3x3 Sudoku PuzzleSudoku Techniques: How to Solve a 3x3 Sudoku Puzzle
Solve 8 Puzzle
How to
Solve 8 Puzzle
Solve Logic Puzzles
How to
Solve Logic Puzzles
Add One Line to Make 200 AnswerHow to Add One Line to Make 100: Answer Revealed
Solve Kakuro
How to
Solve Kakuro
Play Petals Around the Rose
How to
Play Petals Around the Rose
Play Kenken
How to
Play Kenken
Advertisement

About This Article

wikiHow is a “wiki,” similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, volunteer authors worked to edit and improve it over time. This article has been viewed 46,227 times.
53 votes - 66%
Co-authors: 8
Updated: December 23, 2021
Views: 46,227
Categories: Mathematical Puzzles
Advertisement