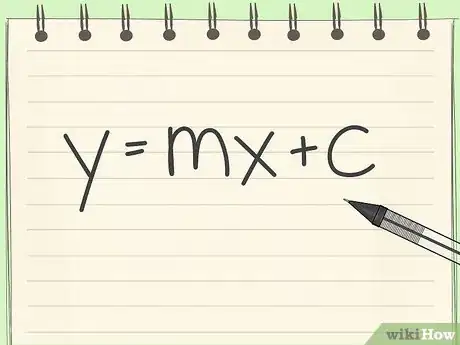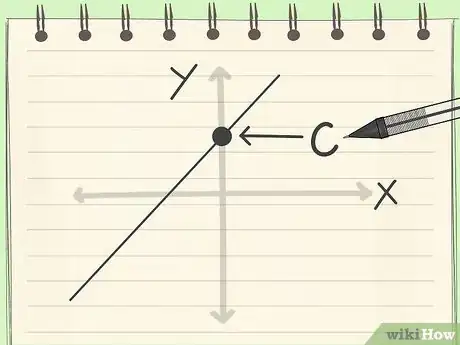How to Quickly Determine the Equation of a Straight Line in a Graph

Last Updated: June 29, 2021

Often, determining the equations of lines on a graph can take a lot of calculation. But with simple straight lines, you need barely any calculations. You can just tell the equation almost immediately by counting the little boxes on the graph paper.

Part 1

Part 1 of 3:

Figuring Out the Equation

1
Know the basic structure for straight line equations. The slope-intercept form will be used commonly here. It is y=mx+c where:^{[1]
X
Expert Source

Grace Imson, MA
Math Instructor, City College of San Francisco
Expert Interview. 1 November 2019.}
- y is the number in relation to the y-axis;
- m is the gradient or slope of the line;
- x is the number in relation to the x-axis;
- and c is the y-intercept.
- To avoid confusion, keep in mind to always have a positive y.
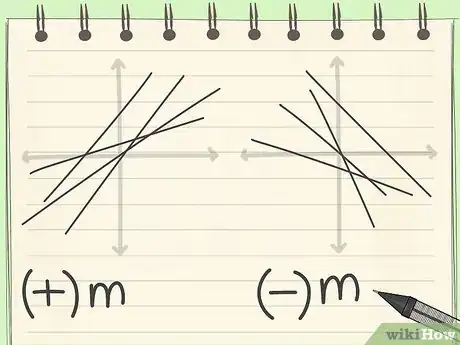
2
Determine if the gradient or m is negative or not. So there are two sides to choose from: y=mx+c or y=-mx+c. If the line goes from top right to bottom left, m is positive. But if the line goes from top left to bottom right, m is negative.

Advertisement
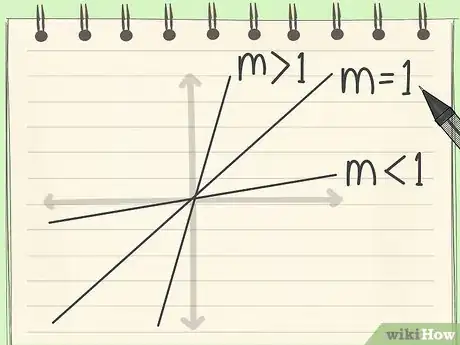
3
Find the gradient. Before you give up and resort to calculating it with numbers, try this simpler way. See if the line is steeper than either y=x or y=-x. If it is steeper, it means m>1. If the line is flatter or less steep, it means m<1.
- Time to count boxes. If m>1, count the vertical boxes for one horizontal box width. Count the number of boxes it takes for the line to reach from one double-integer point (e.g. (2,3) or (5,1); not (5.4, 3) or (1.2, 3.9)) to another double integer point. The number of boxes counted is directly equal to m.
- But if m<1, count the horizontal boxes for a one vertical box width. Let the number of the boxes counted be n. The gradient if m<1 would be one over n or 1/n.
4
Find the y-intercept or c. This is probably the easiest step of all in this how-to article. The y-intercept is the point where the line crosses the y-axis.

Part 2

Part 2 of 3:

Finding the Equation Quickly for Vertical or Horizontal Lines

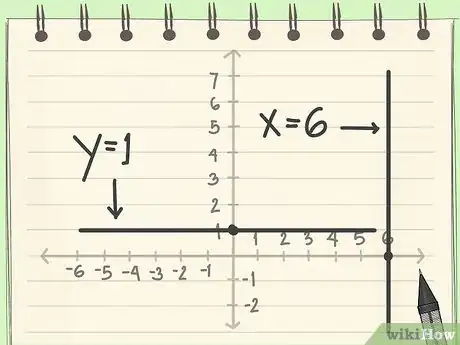
1
Take one good, quick look at the number on the x or y axis. If the line is vertical, look at the x-intercept. If the line is horizontal, look at the y-intercept. The equation for these types of lines are different from the y=mx+c structure.
- Example 1: The line is a vertical line. Thus, we should look at the x-intercept. Looking at it clearly, we could see the number '6'. The equation for this line is x=6. The meaning is that x will always be 6 since the line is straight, so it will stay on 6 and not cross any other axis.
- Example 2: The line is a horizontal line. We should look at the y-intercept. The equation is y=1 because the horizontal line will stay on one forever without crossing the x-axis.
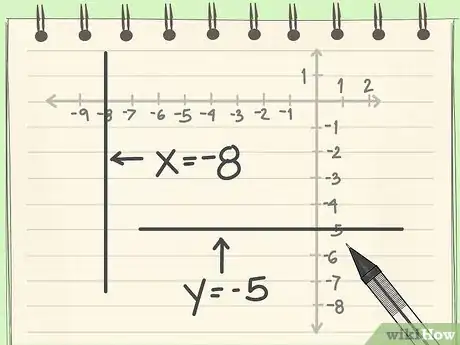
2
Don't forget the lines could be in negative as well.
- Example 3: This line is a vertical line. We should look at x-axis. The line goes with the number '-8'. Thus, the equation to this line is x=-8.
- Example 4: This line is horizontal. Look at the y-axis. The horizontal line aligns with the number '-5'. The equation is y=-5.

Part 3

Part 3 of 3:

Using Examples to Practice More Complicated Lines

1
Practice with some basic non-vertical and non-horizontal examples. Time for something more challenging!
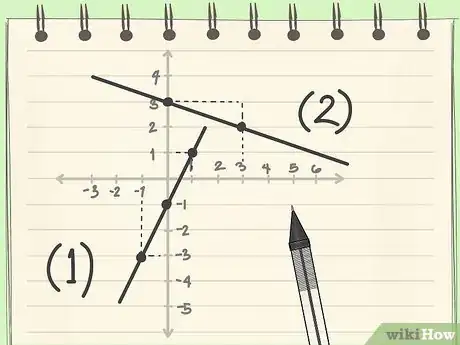
- Example 1: Notice how it takes two vertical blocks to get from one double integer point to another one. Also notice that it is steeper than a simple y=x. We can conclude that the gradient is '2'. So now we've got y=2x. But we're not done yet. We still need to find the y-intercept. Notice that the line crosses the y-axis at '-1' in the y-axis. The equation for this line is indeed y=2x-1.
- Example 2: See that the line goes from top left to bottom right, it means that it has a negative gradient. To reach one double-integer point to another, the number of horizontal blocks is 3 while the number of vertical blocks is 1. It means that the gradient is '-1/3'. The y-intercept is positive 3 as you see the line crossing the y-axis. This line is y=-1/3x+3.
2
Work your way up to harder lines. Study this image. You might have noticed this rule before, but study it to get to know it better. You might also want to look back at some past examples.
- Example 1: Here is a line that is unfamiliar. But look back at the rule above and try to apply the same reasoning with this line. This line has a positive gradient. To get from one double-integer point to another, it vertically goes up 4 blocks and horizontally goes right 3 blocks. Looking back at the rule above, we could determine that this line has a gradient of '4/3'. The y-intercept is 2, so the line is y=4/3x+2.
- Example 2: For this line, we could see that the y-intercept is '0' so we don't need to add anything for c. It has a negative gradient. To get from one double-integer point to another, the number of vertical blocks needed is 3 while the number of horizontal blocks needed is 4. Thus, the equation is y=-3/4x.

Warnings

Make sure that the x to y scale is equal. If it isn't equal, the line would be slightly harder to determine.

⧼thumbs_response⧽
Helpful 0 Not Helpful 0