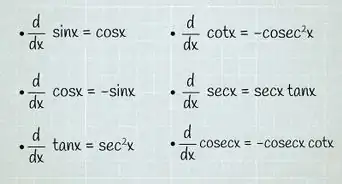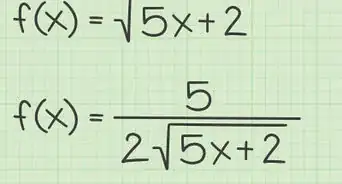How to Pass Calculus

Co-authored by Daron Cam

Last Updated: June 4, 2021 References

Calculus is a class that can be quite difficult for students. Calculus II, in particular, is notorious for being a weed-out class. The key to success in calculus, much like any other technical class like physics or microeconomics, is to understand, deep down, what every topic is getting at.

The truth is, there is no advice that will apply to everyone to acquire the insight needed to solve problems. Intuition seems to work slightly differently for everyone. But this article will attempt to help you in gaining that intuition so you can succeed in this class.

Part 1

Part 1 of 4:

General Guidelines

1
Read the syllabus. It details what concepts you will be learning, what part of the book the material corresponds to, when you have homework, quizzes, and exams, and how much everything is worth.^{[1]
X
Research source}
- The first day of class, add all of the homework due dates and quizzes/exams to your calendar.
- Look for the grade/GPA^{[2]
  X
  Research source} thresholds - the lowest percentage for an A is what you should aim to stay above. Because calculus, especially Calculus II, can be difficult, there may be a curve that depends on the professor and how well your peers do. If the professor doesn't elaborate on his grading, ask.
- Some professors may also have a "course overview" with the syllabus, with textbook sections that correspond. Take a glance at that to get a general picture of what the course will cover.
- Note the topics on related rates, integration techniques, and series - they are often the hardest topics both conceptually and in the amount of algebraic manipulation required to get the answer. Integrating volumes (washers, shells, revolutions) can also be a tricky topic.
2
Talk to students who previously had your professor. Drawing on their knowledge may help you gauge what you need to do to pass the course, such as difficult topics. Of course, what they find difficult may be easy for you, and vice versa, so don't base too much on their opinions. If the professor is the same, they might also know whether there are opportunities for extra credit.

Advertisement
3
Attend office hours! Look on the syllabus to see when office hours are. Most courses also have a TA that helps with grading - they may also have office hours as well. Review the material before you go so you can ask concrete, thoughtful questions instead of just saying, “I’m totally lost.” Professors like students who have made an effort, and it will be obvious to him. Out of all the tips in this section, this may be the most useful.
- This is also an opportunity to get to know your professor. Give some small talk, ask about his life, what he researches, etc. If, at the end of the semester, your grade happens to be on the border, he may be lenient in raising it by that 0.2% to get an A.
- Don't be afraid to ask questions, whether you're in or out of class.^{[3]
  X
  Expert Source
  
  Daron Cam
  Math Tutor
  Expert Interview. 29 May 2020.}
4
Listen and take notes in class. This means writing down definitions, each step of worked examples, important remarks, etc. If going to lectures is how you learn best, you should be able to understand the majority of what goes on your notes because of listening to the professor. If not, ask questions for the things that you absolutely do not understand, since it's likely that other students are wondering too but aren't raising their hands because they don't want to slow the class down.^{[4]
X
Research source}
- Review your notes frequently, especially right after class. Or at least, think about what you just heard. This means while you're doing your homework, at odd times, perhaps even right before class, not just the night before the quiz or test. Take care to not obsess so much over them that it takes over your social life, though.
- As you take notes, try to break down each concept into repeatable steps.^{[5]
  X
  Expert Source
  
  Daron Cam
  Math Tutor
  Expert Interview. 29 May 2020.}
5
Follow example problems in textbooks. Textbooks these days can be obtuse and occasionally very dull. They may be scattered with various theorems or proofs that you may find tough to crack. Very recent textbooks may also have distracting colorful graphics as well.
- Both of those things are not as important as the actual substance. Of course, theorems are important to make the math rigorous, but at the level of calculus, they can be skirted past without much worry. Your professor will probably have more to say on them.
- More importantly, you should do the worked examples as you read through the relevant sections (before the lecture)^{[6]
  X
  Trustworthy Source
  American Psychological Association
  Leading scientific and professional organization of licensed psychologists
  Go to source} so that you understand how the topic is used in a concrete way. If there are graphs or diagrams that go along with them, you should be able to say to yourself, "I know why the graph curves like that."
- If you learn best by reading the textbook, then do so. If the author gives you a query (Why?) to some statement, then you should verify it.
6
Learn how to use your graphing calculator. You probably have a TI-83 or TI-84, or perhaps something newer. Play around with it and get a feel for the basic operations, exponents, trig functions, where you graph, solve matrices, etc. Don't be afraid to ask for help now on how to use it, because you won't get any on the exam.

Part 2

Part 2 of 4:

Reviewing Algebra and Trigonometry

1
Review basic algebra and trigonometry. At the level of calculus, you need to be able to manipulate all the parts of formulas and work with variables. This means adding, subtracting, multiplying, and dividing things to both sides of equations. It also means taking logarithms to both sides to bring down exponents, and vice versa. It also means multiplying by 1 or adding 0 in a clever manner.^{[7]
X
Research source}
- Most students who struggle with calculus fall behind not because of their inability to understand topics in calculus, but because they are not comfortable with algebraic and trig manipulations.
2
Become comfortable with algebraic and trig manipulations in different variables. It is easy to be intimidated by the plethora of letters that aren't $x$ $x$ or $y,$ $y,$ but they are merely symbols for things that you already understand.^{[8]
X
Research source}
- Solving for variables is extremely important. Make sure that you can solve equations like ${\frac {1}{A}}+{\frac {B}{C}}+D^{E}=F$ ${\frac {1}{A}}+{\frac {B}{C}}+D^{{E}}=F$ for any of the six variables in terms of the other ones. To start you off, the answer for $A$ $A$ is given below. Notice there aren't any numbers - this is what we mean by "solve in terms of" the other variables.
  - $A={\frac {1}{F-{\frac {B}{C}}-D^{E}}}$
- Below is another example that you should practice with.
  - $A^{2}-{\frac {BC^{2}}{\sqrt {D}}}=\ln {F}$
- In each result, you should be able to answer when they are undefined. Anything in square roots should be non-negative, anything in the denominator cannot be zero, the domain of the logarithmic function consists of only positive numbers, etc.
3
Review how to solve quadratic equations and how to factor equations. They are essential skills in calculus. You should recognize these things not just in the way that they were originally presented, but be able to recognize equations like $e^{2x}-2e^{x}-6=0$ $e^{{2x}}-2e^{{x}}-6=0$ as quadratic as well (quadratic in $e^{x}$ $e^{{x}}$ - this also means, solve it! There is only one solution. Why?). Furthermore, you should be comfortable with completing the square.
- Equations like ${\frac {1}{x}}+{\frac {2}{x^{2}}}-4=0$ should be easy to solve. The quadratic formula should be drilled into you from past algebra classes.
4
Be sure that you are absolutely comfortable with manipulations that involve exponents and logarithms. In the examples above, you had to use these manipulations to solve for some variables. They were less often used in algebra class, but that just means they are even more important to be familiar with.
- For example, you should be able to solve for exponents in equations like $e^{x}-5=0$ very easily.
- This also means that exponent and log properties are extremely important. When these functions show up, you know these properties are going to be used.
- The exponential function is ubiquitous in calculus because of the unique property that it is its own derivative. This alone means that you have to be comfortable with manipulating exponential functions.
5
Be sure that you are comfortable with trigonometric functions.^{[9]
X
Research source} Functions, identities, and their manipulations are used extensively. You need not memorize all of the identities - they are readily available online - but you should know their domains, ranges, signs (when cosine is positive and negative, for example), and be able to find all solutions to equations like $\sin x=\cos x.$ $\sin x=\cos x.$ Sine, cosine, and tangent are going to be encountered far more often than the others, so you should especially be familiar with them.
- Don't be nervous if not all of the things in this section are second-hand to you. Do some practice problems and check your answers. Each time you do so, you are practicing your manipulation skills.

Part 3

Part 3 of 4:

Some More Advice

1
Do the homework. The key to all STEM classes is practice. This cannot be understated. Do all the problems, and make sure you are doing them correctly by checking your answers after you have attempted it at least once. Go to office hours if you have trouble with a problem. If there is one problem that just cannot be done, do something else - listen to music, go to the gym, text your friends - do something other than math, then come back to it and try again.
- Try to do homework problems as close as you can to a quiz that covers it. This often means that homework will be done the day before it is due. Try to start in the afternoon - you may end up finishing in the evening or into the night. This may be unnerving, but homework is the best practice for doing well on quizzes and exams, and it is not worth the extra two days - two days that you can easily forget half the material for the quiz.
2
Do more practice problems than what’s assigned. To do really well, don't just do the assigned homework; do all the problems.^{[10]
X
Research source} Most textbooks have answers to odd-numbered problems, so do the even-numbered problems as well. If you are strapped for time such that an essay in another class is more important, try to do a few problems every day instead of an entire problem set.
- As you do these problems, you should be consulting your notes, peers, WolframAlpha, etc. less and less often. Remember, they cannot help you on the exam in any way while you are taking it.
3
Look up videos and webpages on these topics. Videos on YouTube especially are a great resource for visualizations that can't be done in a book or on a chalkboard. It is a great way to get an intuitive feel for how things work. Wikipedia pages are also a great resource, though beware that the articles can quickly become very dense.

Part 4

Part 4 of 4:

Passing the Exams

1
Make sure if you can use a cheat sheet on the exam. Some professors allow students to bring a notecard or even a page of notes. Try to take advantage of that so you don't waste time memorizing identities or integrals. Try not to write down things like integrals of even and odd functions over symmetric boundaries, or what to do when you're integrating a product of two functions, or how to implicitly differentiate - those are things that you should know before going in. A general guideline is that the more you find you have to write down (or the smaller your handwriting), the less you probably understand the material.
- If not, then make some time to memorize the important integrals. Memorization is less important in STEM classes, but some calculus classes are relentless with integration, and it may be needed. Ask your professor if such integrals will be on the test. Again, the most important integrals will be used over and over again when doing u-substitution or integration by parts. Since calculus builds on itself, if no notes are allowed and you don't have them in your head when the time comes, you will be in trouble.
- For example, you want to be thinking, "how do I solve $\int x\sin(x^{2})\mathrm {d} x$ by u-sub," not "what was the antiderivative of $\sin u$ again."
2
Form a study group. Get together with some friends or classmates and meet at least once a week to do homework and study for tests and quizzes. Working through material with other people is very helpful because they might understand something that you are unsure of. On the flip side, you might be able to explain something to them that they don’t understand. The important thing with a study group is that you are productive, so you need to spend time with the right people - study groups can also be very unproductive and detract from your success in the class, even if they are your best friends.^{[11]
X
Research source}
- Try to study with people who understand the topic really well.^{[12]
  X
  Expert Source
  
  Daron Cam
  Academic Tutor
  Expert Interview. 29 May 2020.}
3
Teach the material to your friends. Teaching material is the best way to solidify it in your mind. Encourage them to ask questions. Mastery of the material will depend on how many insightful questions you can answer. Be sure that everything you are saying is correct, though. You do not want to have to "unlearn" something because you memorized an identity incorrectly.
- If you find it difficult to explain something easily, go back and review that topic further until it makes sense. Don't stress about explanations too much - if you can easily apply something to problems on a test, that is all that is required.
4
Review problems that you missed. Most professors hand back homework and quizzes and don't take them back. You should be able to explain everything you got wrong - careless mistakes, or more urgently, not knowing where to start on a problem. The material will be in your notes and in the textbook.
- Some professors try to be cheeky and put in questions that many people missed on a later midterm or final. Just go through the problems, discuss them with your friends, look up topics on the Internet if you need further help, and you'll do fine.
5
Eat a good breakfast. Like all tests, don't burn yourself out, and just relax. Eat a banana and walk into the building listening to Mozart, not with equations and concepts swarming in your head.

Expert Q&A

Question

How can I prepare for a calculus test?

Daron Cam
Academic Tutor
Daron Cam is an Academic Tutor and the Founder of Bay Area Tutors, Inc., a San Francisco Bay Area-based tutoring service that provides tutoring in mathematics, science, and overall academic confidence building. Daron has over eight years of teaching math in classrooms and over nine years of one-on-one tutoring experience. He teaches all levels of math including calculus, pre-algebra, algebra I, geometry, and SAT/ACT math prep. Daron holds a BA from the University of California, Berkeley and a math teaching credential from St. Mary's College.

Daron Cam

Academic Tutor

Expert Answer

Take organized notes and highlight all key vocabulary and topics. Also, tools like Kuta software and Khan Academy are great resources you can use to study.
Question

I don't understand the way my teacher explains it. What should I do? He doesn't give a lot of homework.

Community Answer

Spend extra time with this subject, and ask him after school or during school breaks about things you didn't understand/he didn't cover. As for your homework crisis, there are multiple free worksheets online that might help.
Question

Our teacher used topics not in our book and made up tests not related to the homework at all. What should I do to get better grades?

Pimemorized

Top Answerer

To review the topics your teacher has taught, see if you can find the topics online. There are plenty of both videos and worksheets for most calculus topics, so look around and see which one seems most helpful for you. If the tests in particular are hard, go to your teacher and explain your predicament and ask how they recommend reviewing for tests. You can also form a study group with other students in the class to study for your math test together!