Energy of a Bohr Orbit

From Bohr's assumptions, we will now derive a number of important properties of the hydrogen atom from the classical physics. We start by noting the centripetal force causing the electron to follow a circular path is supplied by the Coulomb force. To be more general, we note that this analysis is valid for any single-electron atom. So, if a nucleus has $Z$ protons ($Z=1$ for hydrogen, $Z=2$ for helium, etc.) and only one electron, that atom is called a hydrogen-like atom. 

The spectra of hydrogen-like ions are similar to hydrogen, but shifted to higher energy by the greater attractive force between the electron and nucleus. The magnitude of the centripetal force is $\frac{m_ev^2}{r_n}$, while the Coulomb force is $\frac{Zk_e e^2}{r^2}$. The tacit assumption here is that the nucleus is more massive than the stationary electron, and the electron orbits about it. This is consistent with the planetary model of the atom. Equating these: 

$\displaystyle \frac{m_e v^2}{r} = \frac{Zk_e e^2}{r^2}$

This equation determines the electron's speed at any radius: 

$\displaystyle v = \frac{\sqrt{Zk_e e^2}}{m_e r}$

It also determines the electron's total energy at any radius: 

$\displaystyle E= \frac{1}{2} m_e v^2 - \frac{Z k_e e^2}{r} = - \frac{Z k_e e^2}{2r}$ 

The total energy is negative and inversely proportional to $r$. This means that it takes energy to pull the orbiting electron away from the proton. For infinite values of $r$, the energy is zero, corresponding to a motionless electron infinitely far from the proton.

Now, here comes the Quantum rule: As we saw in the previous module, the angular momentum $L = m_e r v$ is an integer multiple of $\hbar$: 

$m_e v r = n \hbar$

Substituting the expression in the equation for speed above gives an equation for $r$ in terms of $n$: 

$\sqrt{Zk_e e^2 m_e r} = n \hbar$

The allowed orbit radius at any n is then: 

$\displaystyle r_n = {n^2\hbar^2\over Zk_e e^2 m_e}$

The smallest possible value of $r$ in the hydrogen atom is called the Bohr radius and is equal to 0.053 nm. The energy of the $n$-th level for any atom is determined by the radius and quantum number: 

$\displaystyle E = -\frac{Zk_e e^2}{2r_n } = - \frac{ Z^2(k_e e^2)^2 m_e }{2\hbar^2 n^2} \approx \frac{-13.6Z^2}{n^2}\mathrm{eV}$

Using this equation, the energy of a photon emitted by a hydrogen atom is given by the difference of two hydrogen energy levels: 

$\displaystyle E=E_i-E_f=R \left( \frac{1}{n_{f}^2} - \frac{1}{n_{i}^2} \right)$

Which is the Rydberg formula describing all the hydrogen spectrum and $R$ is the Rydberg constant. Bohr's model predicted experimental hydrogen spectrum extremely well.

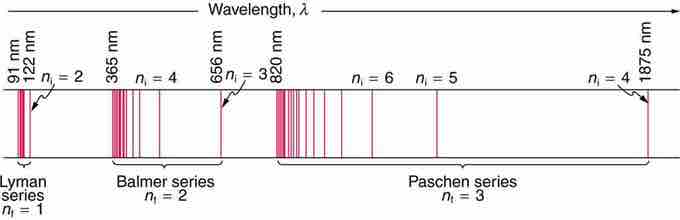
Fig 1

A schematic of the hydrogen spectrum shows several series named for those who contributed most to their determination. Part of the Balmer series is in the visible spectrum, while the Lyman series is entirely in the UV, and the Paschen series and others are in the IR. Values of nf and ni are shown for some of the lines.