Also called the infinite square well problem, the particle in a box is one of the very few problems in quantum mechanics that can be solved without approximations.
The solution to the particle in a box can be found by solving the Schrödinger equation:
The potential function (V) is time-independent, while the wavefunction itself is time- dependent.
The infinite square well is defined by a potential function in which the potential
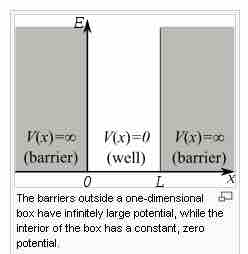
The potential well
Energy and position relationships of the particle in a box. Inside the box the potential V(x) is zero. Outside the box the potential energy is infinite.
Separating the variables reduces the problem to one of simply solving the spatial part of the equation:
E represents the possible energies that can describe the system. The above equation establishes a direct relationship between the second derivative of the the wave function and the kinetic energy of the system. The best way to visualize the time-independent Schrödinger equation is as a stationary snapshot of a wave at particular moment in time.
No forces act on the particle inside of a box, which means that the part of the wave function between 0 and L can oscillate through space and time with the same form as a free particle:
B and A are arbitrary complex numbers. The frequency of the oscillations through space and time are given by the wave number,
The size or amplitude of the wave function at any point determines the probability of finding the particle at that location, as given by the equation:
The wavefunction must vanish everywhere beyond the edges of the box, as the potential outside of the box is infinite. Furthermore, the amplitude of the wavefunction also may not "jump" abruptly from one point to the next. These two conditions are only satisfied by wavefunctions with the form:
because wavefunctions based on sine waves will have Ψ(x) = 0 values when x = o and x = L, while those wave functions which include cosine terms will not.
where n = {1,2,3,4...} and L is the size of the box . Negative values are neglected, since they give wavefunctions identical to the positive solutions except for a physically unimportant sign change. Finally, the unknown constant may be found by normalizing the wavefunction so that the total probability density of finding the particle in the system is 1.
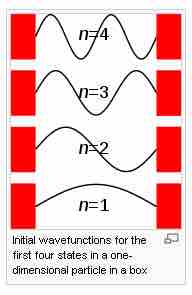
Solutions to the particle in a box problem
The first four solutions to the one dimensional particle in a box. Note that just like a guitar string, the solutions to the particle in a box problem are constrained to those wavefunctions that anchor the amplitude at the walls of the box as zero.
Normalizing, we get
A may be any complex number with absolute value
When treated as a probability density, the square of the wave function (Ψ2) describes the probability of finding the particle at a given point and at a given time. Four conditions, proposed by Max Born, must be met for this to be true:
- The wave function must be single-valued.
- The wave function must be "square integrable."
- The wave function must be continuous everywhere.
- The first derivative of the wave function must be continuous.