In electrochemistry, the Nernst equation can be used, in conjunction with other information, to determine the reduction potential of a half-cell in an electrochemical cell. It can also be used to determine the total voltage, or electromotive force, for a full electrochemical cell. It is named after the German physical chemist who first formulated it, Walther Nernst.
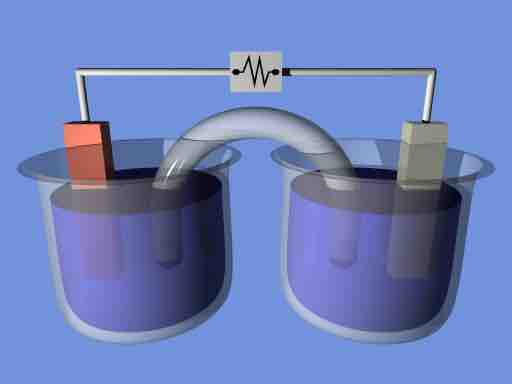
Electrochemical cell
Schematic of an electrochemical cell.
The Nernst equation gives a formula that relates the electromotive force of a nonstandard cell to the concentrations of species in solution:
In this equation:
- E is the electromotive force of the non-standard cell
- Eo is the electromotive force of the standard cell
- n is the number of moles of electrons transferred in the reaction
ln Q is the natural log of
Example
Find the cell potential of a galvanic cell based on the following reduction half-reactions where [Ni2+] = 0.030 M and [Pb2+] = 0.300 M.
Ni2+ + 2 e- → Ni, E0 = -0.25 V
Pb2+ + 2 e- → Pb, E0 = -0.13 V
First, find the electromotive force for the standard cell, which assumes concentrations of 1 M.
In order for this reaction to run spontaneously (positive Eo cell) the nickel must be oxidized and therefore its reaction needs to be reversed. The added half-reactions with the adjusted E0 cell are:
The number of moles of electrons transferred is 2 and Q is