What Does pH Mean in a Buffer?
In chemistry, pH is a measure of the hydrogen ion (H+) concentration in a solution. The pH of a buffer can be calculated from the concentrations of the various components of the reaction. The balanced equation for a buffer is:
The strength of a weak acid is usually represented as an equilibrium constant. The acid-dissociation equilibrium constant (Ka), which measures the propensity of an acid to dissociate, for the reaction is:
The greater [H+] x [A-] is than [HA], the greater the value of Ka, the more the formation of H+ is favored, and the lower the pH of the solution.
ICE Tables: A Useful Tool For Solving Equilibrium Problems
ICE (Initial, Change, Equilibrium) tables are very helpful tools for understanding equilibrium and for calculating the pH of a buffer solution. They consist of using the initial concentrations of reactants and products, the change they undergo during the reaction, and their equilibrium concentrations. Consider, for example, the following problem:
Calculate the pH of a buffer solution that initially consists of 0.0500 M NH3 and 0.0350 M NH4+. (Note: Ka for NH4+ is 5.6 x 10-10). The equation for the reaction is as follows:
We know that initially there is 0.0350 M NH4+ and 0.0500 M NH3. Before the reaction occurs, no H+ is present so it starts at 0.
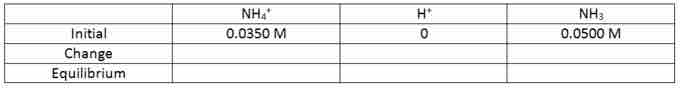
ICE table - initial
ICE table for the buffer solution of NH4+ and NH3 with the starting concentrations.
During the reaction, the NH4+ will dissociate into H+ and NH3. Because the reaction has a 1:1 stoichiometry, the amount that NH4+ loses is equal to the amounts that H+ and NH3 will gain. This change is represented by the letter x in the following table.

ICE table - change
Describes the change in concentration that occurs during the reaction.
Therefore the equilibrium concentrations will look like this:
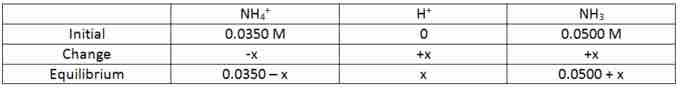
ICE table - equilibrium
Describes the final concentration of the reactants and products at equilibrium.
Apply the equilibrium values to the expression for Ka.
Assuming x is negligible compared to 0.0500 and 0.0350 the equation is reduced to:
Solving for x (H+):
x = [H+] = 3.92 x 10-10
pH = -log(3.92 x 10-10)
pH = 9.41