A solid of revolution is a solid figure obtained by rotating a plane curve around some straight line (the axis) that lies on the same plane . Here, we will study how to compute volumes of these objects. Two common methods for finding the volume of a solid of revolution are the disc method and the shell method of integration. To apply these methods, it is easiest to draw the graph in question; identify the area that is to be revolved about the axis of revolution; determine the volume of either a disc-shaped slice of the solid, with thickness

A Volume of Revolution
A solid formed by rotating a curve around an axis.
Disc Method
The disc method is used when the slice that was drawn is perpendicular to the axis of revolution; i.e. when integrating parallel to the axis of revolution. The volume of the solid formed by rotating the area between the curves of
If
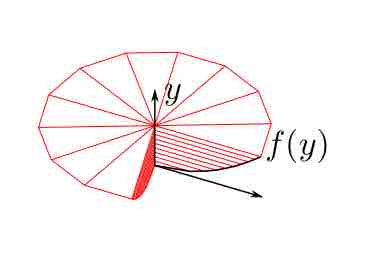
Disc Integration
Disc integration about the
The method can be visualized by considering a thin horizontal rectangle at
where
An infinite sum of the discs between
Shell Method
The shell method is used when the slice that was drawn is parallel to the axis of revolution; i.e. when integrating perpendicular to the axis of revolution. The volume of the solid formed by rotating the area between the curves of
If

Shell Integration
The integration (along the
The method can be visualized by considering a thin vertical rectangle at