Cylindrical Shells

Shell integration (also called the shell method) is a means of calculating the volume of a solid of revolution when integrating perpendicular to the axis of revolution . (When integrating parallel to the axis of revolution, you should use the disk method. ) While less intuitive than disk integration, it usually produces simpler integrals. Intuitively speaking, part of the graph of a function is rotated around an axis, and is modeled by an infinite number of cylindrical shells, all infinitely thin.

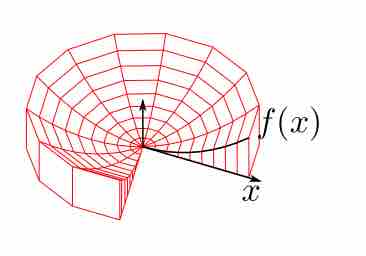
The Shell Method

Calculating volume using the shell method. Each segment located at $x$, between $f(x)$and the $x$-axis, gives a cylindrical shell after revolution around the vertical axis.

The idea is that a "representative rectangle" (used in the most basic forms of integration, such as $\int x \,dx$) can be rotated about the axis of revolution, thus generating a hollow cylinder with infinitesimal volume. Integration, as an accumulative process, can then calculate the integrated volume of a "family" of shells (a shell being the outer edge of a hollow cylinder), giving us the total volume.

The volume of the solid formed by rotating the area between the curves of $f(x)$ and $g(x)$ and the lines $x=a$ and $x=b$ about the $y$-axis is given by:

$\displaystyle{V = 2\pi \int_a^b x \left | f(x) - g(x) \right | \,dx}$

In the integrand, the factor $x$ represents the radius of the cylindrical shell under consideration, while  is equal to the height of the shell. Therefore, the entire integrand, $2\pi x \left | f(x) - g(x) \right | \,dx$, is nothing but the volume of the cylindrical shell. By adding the volumes of all these infinitely thin cylinders, we can calculate the volume of the solid formed by the revolution.

The volume of solid formed by rotating the area between the curves of $f(y)$ and and the lines $y=a$ and $y=b$ about the $x$-axis is given by:

$\displaystyle{V = 2\pi \int_a^b x \left | f(y) - g(y) \right | \,dy}$