In calculus, the second derivative test is a criterion for determining whether a given critical point of a real function of one variable is a local maximum or a local minimum using the value of the second derivative at the point.
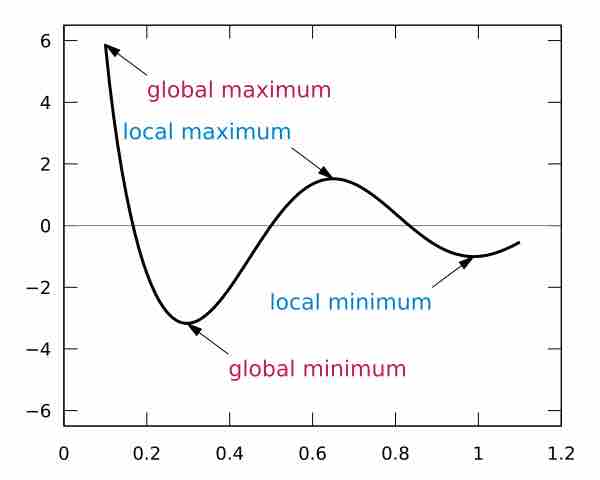
Maxima and Minima
Telling whether a critical point is a maximum or a minimum has to do with the second derivative. If it is concave-up at the point, it is a minimum; if concave-down, it is a maximum.
The test states: if the function
If
If
If
In the latter case, Taylor's Theorem may be used to determine the behavior of
Proof of the Second Derivative Test:
Suppose we have
Thus, for a sufficiently small
which means that
A related but distinct use of second derivatives is to determine whether a function is concave up or concave down at a point. It does not, however, provide information about inflection points. Specifically, a twice-differentiable function