Parametric Surfaces and Surface Integrals

Parametric Surface

A parametric surface is a surface in the Euclidean space $R^3$ which is defined by a parametric equation with two parameters: $\vec r: \Bbb{R}^2 \rightarrow \Bbb{R}^3$. Parametric representation is the most general way to specify a surface. Surfaces that occur in two of the main theorems of vector calculus, Stokes' theorem and the divergence theorem, are frequently given in a parametric form. The curvature and arc length of curves on the surface can both be computed from a given parametrization.

Examples

• The simplest type of parametric surfaces is given by the graphs of functions of two variables: $z=f(x,y)$; $\vec{r}(x,y)=(x,y,f(x,y))$.
• Using the spherical coordinates, the unit sphere can be parameterized by $\vec r(\theta,\phi) = (\cos\theta \sin\phi, \sin\theta \sin \phi, \cos\phi), 0 \leq \theta < 2\pi, 0 \leq \phi \leq \pi$.
• The straight circular cylinder of radius $R$ about the $x$-axis has the following parametric representation: $\vec{r}(x,\phi)=(x,R \cos \phi, r \sin \phi)$.

The same surface admits many different parametrizations. For example, the coordinate $z$-plane can be parametrized as $\vec r(u,v)=(au+bv,cu+dv, 0)$ for any constants $a$, $b$, $c$, $d$ such that $ad - bc \neq 0$, i.e. the matrix $\begin{bmatrix}a & b\\ c & d\end{bmatrix}$ is invertible.

Surface integral

A surface integral is a definite integral taken over a surface . It can be thought of as the double integral analog of the line integral. Given a surface, one may integrate over its scalar fields (that is, functions which return scalars as values), and vector fields (that is, functions which return vectors as values). Surface integrals have many applications in physics, particularly within the classical theory of electromagnetism. We will study surface integral of vector fields and related theorems in the following atoms.

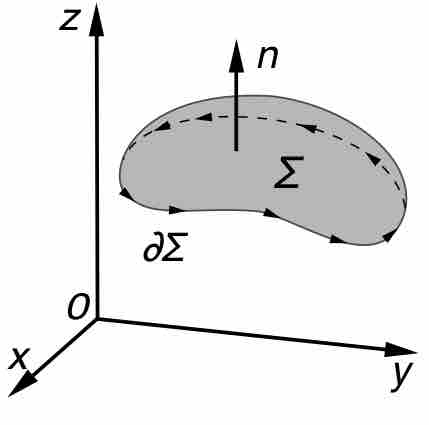
Kelvin-Stokes' Theorem

An illustration of the Kelvin–Stokes theorem, with surface $\Sigma$, its boundary $\partial$, and the "normal" vector $\mathbf{n}$.