A partial derivative of a function of several variables is its derivative with respect to one of those variables, with the others held constant (as opposed to the total derivative, in which all variables are allowed to vary). Partial derivatives are used in vector calculus and differential geometry. The partial derivative of a function f with respect to the variable x is variously denoted by
Suppose that f is a function of more than one variable. For instance,
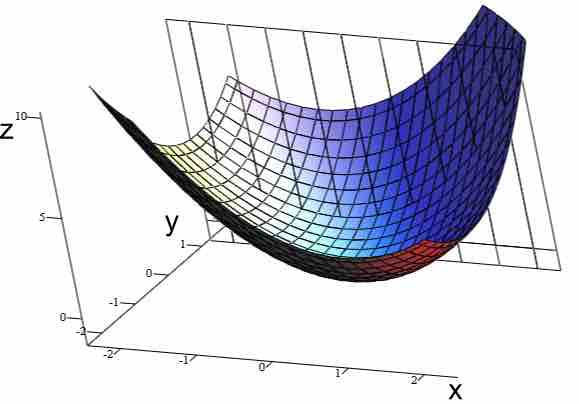
Graph of $z = x^2 + xy + y^2$
For the partial derivative at
To find the slope of the line tangent to the function at
So at
at the point
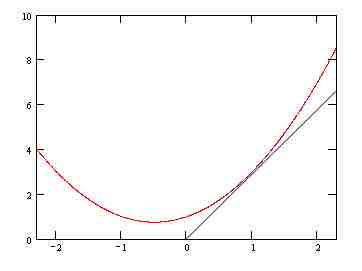
Graph of $z = x^2 + xy + y^2$ at $y=1$
A slice of the graph at
Formal Definition
Like ordinary derivatives, the partial derivative is defined as a limit. Let