The multiple integral is a type of definite integral extended to functions of more than one real variable—for example,
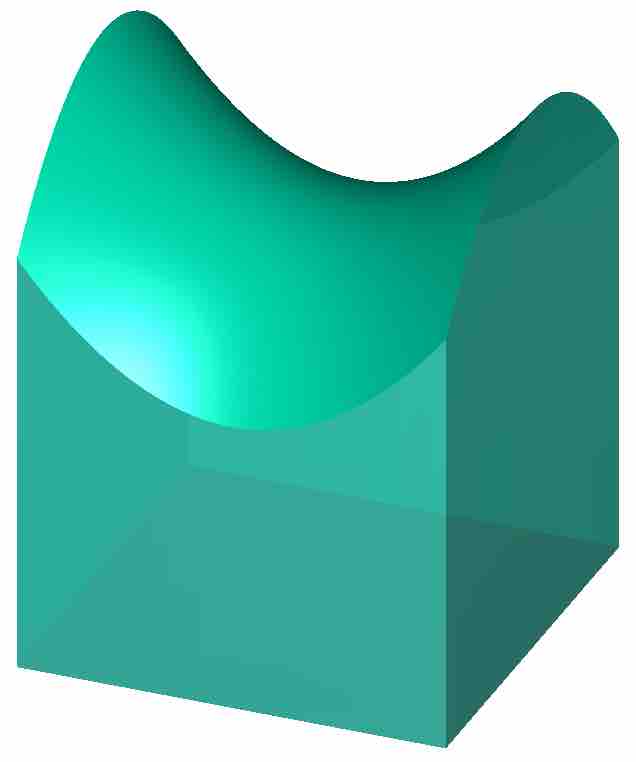
Volume to be Integrated
Double integral as volume under a surface
Double Integrals Over Rectangles
Double integrals over rectangular regions are straightforward to compute in many cases. For a rectangular region
Here, we exchanged the order of the integration, assuming that
Example
Let us assume that we wish to integrate a multivariable function
Formulating the double integral , we first evaluate the inner integral with respect to
We then integrate the result with respect to
We could have computed the double integral starting from the integration over