Double Integrals in Polar Coordinates

In $R^2$, if the domain has a cylindrical symmetry and the function has several particular characteristics, you can apply the transformation to polar coordinates, which means that the generic points $P(x, y)$ in Cartesian coordinates switch to their respective points in polar coordinates. This allows one to change the shape of the domain and simplify the operations.

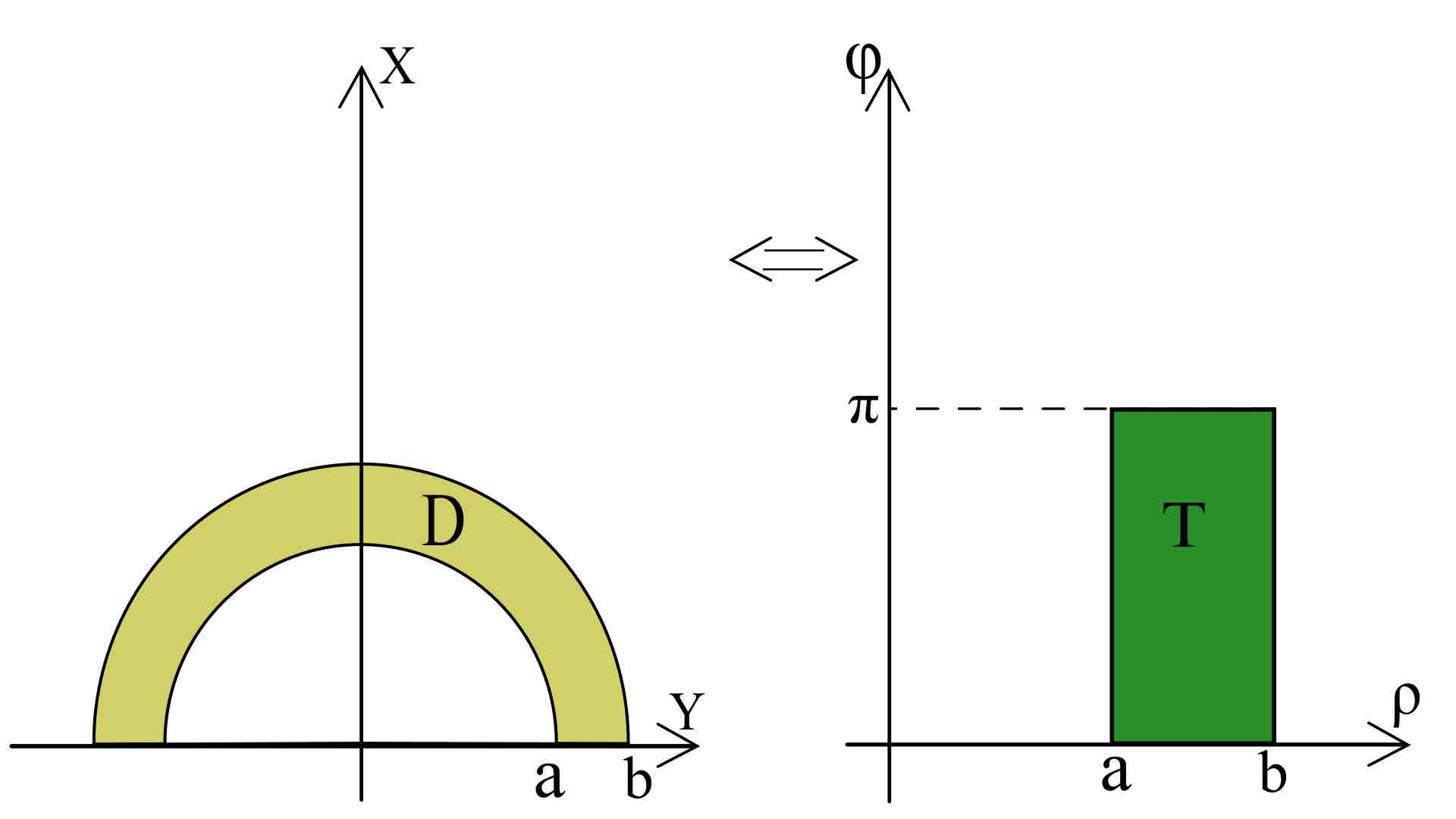
Transformation to Polar Coordinates

This figure illustrates graphically a transformation from cartesian to polar coordinates

Change of variable

The polar coordinates $r$ and $\varphi$ can be converted to the Cartesian coordinates $x$ and $y$ by using the trigonometric functions sine and cosine:

$x = r \cos \varphi \, \\ y = r \sin \varphi \,$

The Cartesian coordinates $x$ and $y$ can be converted to polar coordinates $r$ and $\varphi$ with $r \geq 0$ and $\varphi$ in the interval $(−\pi, \pi]$:

$r = \sqrt{x^2 + y^2} \\ \varphi = \tan ^{-1} (\frac yx)$

The fundamental relation to make the transformation is as follows:

$f(x,y) \rightarrow f(\rho \cos \phi,\rho \sin \phi )$

Examples

• Given the function $f(x,y) = x + y$ and applying the transformation, one obtains $f(\rho, \phi) = \rho \cos \phi + \rho \sin \phi = \rho(\cos \phi + \sin \phi )$.
• Given the function $f(x,y) = x^2 + y^2$, one can obtain $f(\rho, \phi) = \rho^2 (\cos^2 \phi + \sin^2 \phi) = \rho^2$ using the Pythagorean trigonometric identity, which is very useful to simplify this operation. Particularly in this case, you can see that the representation of the function f became simpler in polar coordinates. This is the case because the function has a cylindrical symmetry. In general, the best practice is to use the coordinates that match the built-in symmetry of the function.

Integrals in Polar Coordinates

The Jacobian determinant of that transformation is the following: 

$\displaystyle{\frac{\partial (x,y)}{\partial (\rho, \phi)}} = \begin{vmatrix} \cos \phi & - \rho \sin \phi \\ \sin \phi & \rho \cos \phi \end{vmatrix} = \rho$

which has been obtained by inserting the partial derivatives of $x = \rho \cos(\varphi)$, $y = \rho \sin(\varphi)$ in the first column with respect to $\rho$ and in the second column with respect to $\varphi$, so the $dx \, dy$ differentials in this transformation become $\rho \,d \rho \,d\varphi$. Once the function is transformed and the domain evaluated, it is possible to define the formula for the change of variables in polar coordinates:

$\iint_D f(x,y)dx \, dy = \iint_T f(\rho \cos \varphi, \rho \sin \varphi)\rho$

Example

Integrate the function $f(x,y) = x$ over the domain:

$D = \{ x^2 + y^2 \le 9, \ x^2 + y^2 \ge 4, \ y \ge 0 \}$

From $f(x,y) = x \longrightarrow f(\rho,\phi) = \rho \cos \phi$, 

$\begin{aligned} \iint_D x \, dx\, dy &= \iint_T \rho \cos \phi \rho \, d\rho\, d\phi \\ &= \int_0^\pi \int_2^3 \rho^2 \cos \phi \, d \rho \, d \phi \\ &= \int_0^\pi \cos \phi \ d \phi \left[ \frac{\rho^3}{3} \right]_2^3 \\ &= \left[ \sin \phi \right]_0^\pi \ \left(9 - \frac{8}{3} \right) = 0 \end{aligned}$