Even and Odd Definitions
Functions can be classified as "odd" or "even" based on their composition. These labels correlate with symmetry properties of the function.
The terms "odd" and "even" can only be applied to a limited set of functions. For a function to be classified as one or the other, it must have an additive inverse. Therefore, it must have a number that, when added to it, equals
Oftentimes, the parity of a function will reveal whether it is odd or even. For example, the function
Even Functions
Even functions are algebraically defined as functions in which the following relationship holds for all values of
To check if a function is even, any
Example 1: Is the function $f(x)=x^4+2x$ even?
Remember the degree of the function, in this case a
First, perform an algebraic check: Substitute a value for
Therefore
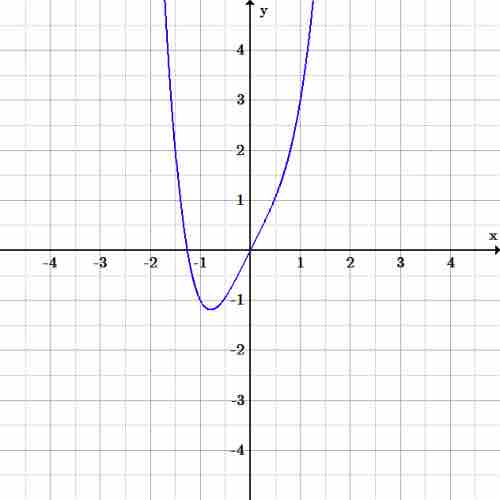
Symmetry: even function?
The function
We can confirm this graphically: functions that satisfy the requirements of being even are symmetric about the
Odd Functions
Odd functions are algebraically defined as functions in which the following relationship holds true for all values of
This relationship can also be expressed as:
To check if a function is odd, the negation of the function (be sure to negate all terms of the function) must yield the same output as substituting the value
Example 2: Is the function $f(x)=x^3-9x$ odd?
Algebraic check: Does
Therefore
Graphical check: Functions that satisfy the requirements of being odd are symmetric with respect to the origin. In other words, rotating the graph

Symmetry: odd function?
The function,