Spring force is conservative force, given by the Hooke's law : F = -kx, where k is spring constant, measured experimentally for a particular spring and x is the displacement . We would like to obtain an expression for the work done to the spring. From the conservation of mechanical energy (Check our Atom on "Conservation of Mechanical Energy), the work should be equal to the potential energy stored in spring. The displacement x is usually measured from the position of "neutral length" or "relaxed length" - the length of spring corresponding to situation when spring is neither stretched nor compressed. We shall identify this position as the origin of coordinate reference (x=0).
Hooke's Law
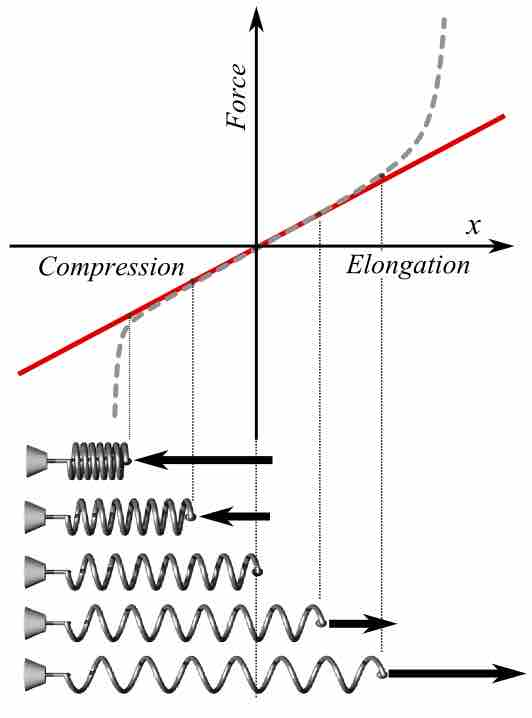
Plot of applied force F vs. elongation X for a helical spring according to Hooke's law (solid line) and what the actual plot might look like (dashed line). Red is used extension, blue for compression. At bottom, schematic pictures of spring states corresponding to some points of the plot; the middle one is in the relaxed state (no force applied).
Let x = 0 and x = xf (>0) be the initial and final positions of the block attached to the string. As the block slowly moves, we do work W on the spring:
If the block is gently released from the stretched position (x = xf), the stored potential energy in the spring will start to be converted to the kinetic energy of the block, and vice versa. Neglecting frictional forces, Mechanical energy conservation demands that, at any point during its motion,
From the energy conservation, we can estimate that, by the time the block reaches x=0 position, its speed will be