An isothermal process is a change of a system, in which the temperature remains constant: ΔT = 0. This typically occurs when a system is in contact with an outside thermal reservoir (heat bath), and the change occurs slowly enough to allow the system to continually adjust to the temperature of the reservoir through heat exchange. In contrast, an adiabatic process is where a system exchanges no heat with its surroundings (Q = 0). (See our atom on "Adiabatic Process. ") In other words, in an isothermal process, the value ΔT = 0 but Q ≠ 0, while in an adiabatic process, ΔT ≠ 0 but Q = 0.
Ideal Gas in an Isothermal Process
For an ideal, the product of pressure and volume (PV) is a constant if the gas is kept at isothermal conditions. (This is historically called Boyle's law. ) However, the cases where the product PV is an exponential term, does not comply. The value of the constant is nRT, where n is the number of moles of gas present and R is the ideal gas constant. In other words, the ideal gas law PV = nRT applies. This means that
holds. The family of curves generated by this equation is shown in . Each curve is called an isotherm.
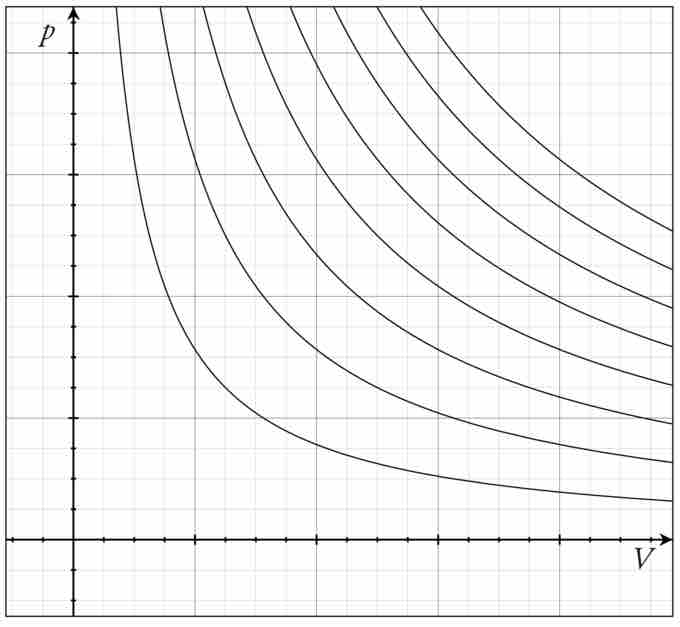
Isotherms of an Ideal Gas
Several isotherms of an ideal gas on a PV diagram.
Calculation of Work
In thermodynamics, the work involved when a gas changes from state A to state B is simply
For an isothermal, reversible process, this integral equals the area under the relevant pressure-volume isotherm, and is indicated in blue in for an ideal gas. Again, P = nRT / V applies and with T being constant (as this is an isothermal process), we have
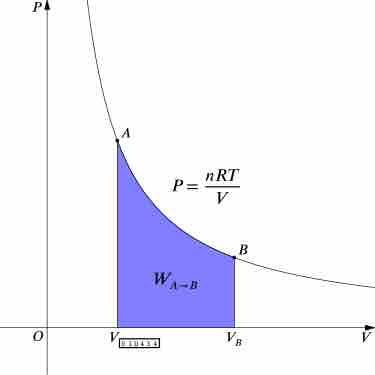
Work Done by Gas During Expansion
The blue area represents "work" done by the gas during expansion for this isothermal change.
It is also worth noting that, for many systems, if the temperature is held constant, the internal energy of the system also is constant, and so