Problem-Solving Techniques

Problem-Solving Strategy For Rotational Kinematics

When solving problems on rotational kinematics:

• Examine the situation to determine that rotational kinematics (rotational motion) is involved. Rotation must be involved, but without the need to consider forces or masses that affect the motion.
• Identify exactly what needs to be determined in the problem (identify the unknowns). A sketch of the situation is useful.
• Make a list of what is given or can be inferred from the problem as stated (identify the knowns).
• Solve the appropriate equation or equations for the quantity to be determined (the unknown). It can be useful to think in terms of a translational analog because by now you are familiar with such motion .
• Substitute the known values along with their units into the appropriate equation, and obtain numerical solutions complete with units. Be sure to use units of radians for angles.
• Check your answer to see if it is reasonable: Does your answer make sense?

Example

Suppose a large freight train accelerates from rest, giving its 0.350 m radius wheels an angular acceleration of 0.250 rad/s². After the wheels have made 200 revolutions (assume no slippage): (a) How far has the train moved down the track? (b) What are the final angular velocity of the wheels and the linear velocity of the train?

In part (a), we are asked to find x, and in (b) we are asked to find ω and v. We are given the number of revolutions θ, the radius of the wheels r, and the angular acceleration α.

The distance x is very easily found from the relationship between distance and rotation angle:$\theta =x/r$.

Solving this equation for x yields $x=r\theta $.

Before using this equation, we must convert the number of revolutions into radians, because we are dealing with a relationship between linear and rotational quantities:

$\theta = (200rev)(2\pi rad/1 rev) = 1257 rad$.

Substitute the known values into $x=r\theta $ to find the distance the train moved down the track:

$x = r\theta = (0.350 m)(1257 rad) = 440 m$.

We cannot use any equation that incorporates t to find ω, because the equation would have at least two unknown values. The equation $\omega 2=\omega 02+2$ will work, because we know the values for all variables except ω. Taking the square root of this equation and entering the known values gives

$\omega = \sqrt{0+2(0.250~rad/s^2)(1257~rad)} \\= 25.1~rad/s$

One may find the linear velocity of the train, v, through its relationship to ω:

$v = r\omega = (0.350 m)(25.1 rad/s) = 8.77 m/s$

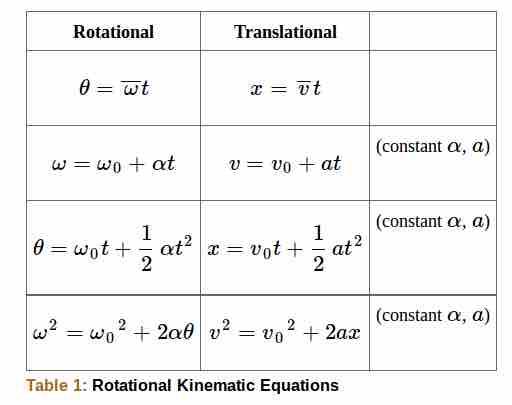
Equation list

Rotational and translational kinematic equations.