In classical mechanics, linear momentum, or simply momentum (SI unit kg m/s, or equivalently N s), is the product of the mass and velocity of an object. Mathematically it is stated as:
(Note here that p and v are vectors. ) Like velocity, linear momentum is a vector quantity, possessing a direction as well as a magnitude. Linear momentum is particularly important because it is a conserved quantity, meaning that in a closed system (without any external forces) its total linear momentum cannot change.
Because momentum has a direction, it can be used to predict the resulting direction of objects after they collide, as well as their speeds. Momentum is conserved in both inelastic and elastic collisions. (Kinetic energy is not conserved in inelastic collisions but is conserved in elastic collisions. ) It important to note that if the collision takes place on a surface with friction, or if there is air resistance, we would need to account for the momentum of the bodies that would be transferred to the surface and/or air.
Let's take a look at a simple, one-dimensional example: The momentum of a system of two particles is the sum of their momenta. If two particles have masses m1 and m2, and velocities v1 and v2, the total momentum is:
Keep in mind that momentum and velocity are vectors. Therefore, in 1D, if two particles are moving in the same direction, v1 and v2 have the same sign. If the particles are moving in opposite directions they will have opposite signs.
If two particles were moving on a plane we would choose our xy-plane to be on the plane of motion. We can then write the x and y component of the total momentum as:
If the 2D momentum vector is decomposed into two components, the equations for each component are reduced to its 1D equivalents.
Momentum, like energy, is important because it is conserved. "Newton's cradle" shown in is an example of conservation of momentum. As we will discuss in the next concept (on Momentum, Force, and Newton's Second Law), in classical mechanics, conservation of linear momentum is implied by Newton's laws. Only a few physical quantities are conserved in nature. Studying these quantities yields fundamental insight into how nature works.
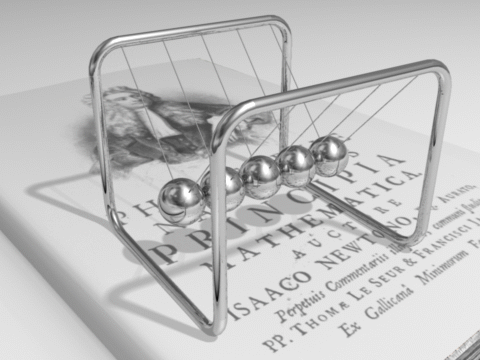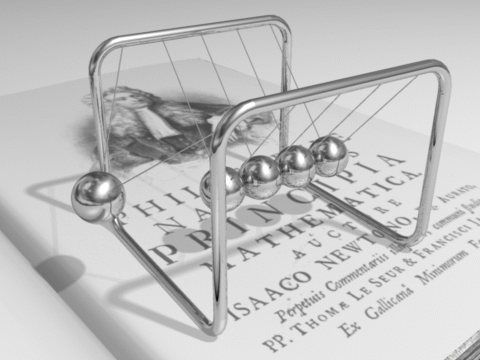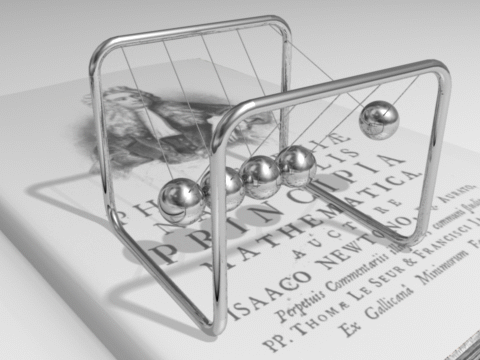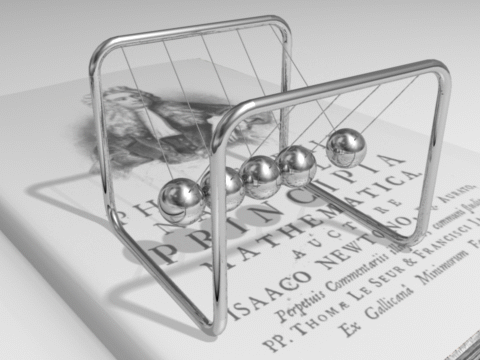
Newton's Cradle
Total momentum of the system (or Cradle) is conserved. (neglecting frictional loss in the system. )