Under standard conditions, water will self-ionize to a very small extent. The self-ionization of water refers to the reaction in which a water molecule donates one of its protons to a neighboring water molecule, either in pure water or in aqueous solution. The result is the formation of a hydroxide ion (OH-) and a hydronium ion (H3O+). The reaction can be written as follows:
This is an example of autoprotolysis (meaning "self-protonating") and it exemplifies the amphoteric nature of water (ability to act as both an acid and a base).
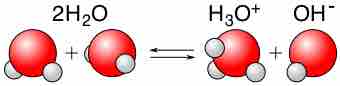
Autoprotolysis of water
The self-ionization of water produces hydronium and hydroxide ions in solution.
The Water Ionization Constant, KW
Note that the self-ionization of water is an equilibrium reaction:
Like all equilibrium reactions, this reaction has an equilibrium constant. Because this is a special equilibrium constant, specific to the self-ionization of water, it is denoted KW; it has a value of 1.0 x 10−14. If we write out the actual equilibrium expression for KW, we get the following:
However, because H+ and OH- are formed in a 1:1 molar ratio, we have:
Now, note the definition of pH and pOH:
If we plug in the above value into our equation for pH, we find that:
Here we have the reason why neutral water has a pH of 7.0; it represents the condition at which the concentrations of H+ and OH- are exactly equal in solution.
pH, pOH, and pKW
We have already established that the equilibrium constant KW can be expressed as:
If we take the negative logarithm of both sides of this equation, we get the following:
However, because we know that pKW = 14, we can establish the following relationship:
This relationship always holds true for any aqueous solution, regardless of its level of acidity or alkalinity. Utilizing this equation is a convenient way to quickly determine pOH from pH and vice versa, as well as to determine hydroxide concentration given hydrogen concentration, or vice versa.