Introduction
In calculus, integration by parts is a theorem that relates the integral of a product of functions to the integral of their derivative and anti-derivative. It is frequently used to find the anti-derivative of a product of functions into an ideally simpler anti-derivative. The rule can be derived in one line by simply integrating the product rule of differentiation.
Theorem of integration by parts
Let's take the functions
or, more compactly,
Proof
Suppose
Integrating both sides with respect to
then applying the fundamental theorem of calculus,
gives the formula for "integration by parts":
Visulization
Let's define a parametric curve by
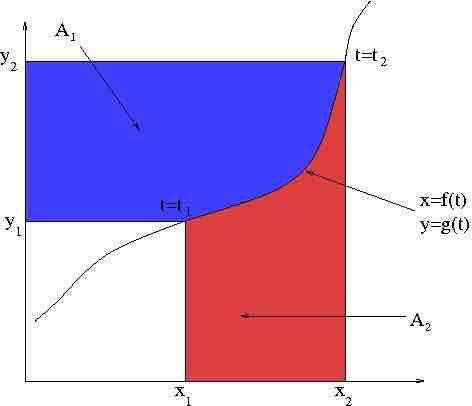
Integration By Parts
Integration by parts may be thought of as deriving the area of the blue region from the total area and that of the red region. The area of the blue region is
Example
In order to calculate
and
then: