Implicit Differentiation

An implicit function is a function that is defined implicitly by a relation between its argument and its value.

If the left hand side of the equation $R(x, y) = 0$ is differentiable and satisfies some mild condition on its partial derivatives at some point $(a, b)$ such that $R(a, b) = 0$, then it defines a function $y = f(x)$ over some interval containing $a$. Geometrically, the graph defined by $R(x, y) = 0$ will overlap locally with the graph of some equation $y = f(x)$.

For most implicit functions, there is no formula which defines them explicitly. However, various numerical methods exist for computing approximately the value of $y$ corresponding to any fixed value of $x$; this allows us to find an explicit approximation to the implicit function.

Implicit differentiation makes use of the chain rule to differentiate implicitly defined functions.

Previously, the functions we have investigated were explicit functions of one variable with respect to another. $y= x^2$ is an explicit function because it shows you exactly what $y$ is with respect to $x$. An implicit function doesn't tell you exactly what $y$ is but still relates $y$ to $x$. For example, $y^2+y=\sin (x)$ is an implicit function because it is very difficult to get a formula for $y$ in terms of only x. However, we can still find the derivative of $y$ with respect to x by using implicit differentiation.

$\displaystyle{2y \cdot \frac{dy}{dx}+\frac{dy}{dx} =\cos(x)=(2y+1)\frac{dy}{dx}}$

Therefore:

$\displaystyle{\frac{dy}{dx}=\frac{\cos(x)}{2y+1}}$

As $y$ can be given as a function of $x$ implicitly rather than explicitly, when we have an equation $R(x, y) = 0$, we may be able to solve it for $y$ and then differentiate. However, sometimes it is simpler to differentiate $R(x, y)$ with respect to $x$ and $y$ and then solve for $\frac{dy}{dx}$.

For example, given the expression $y + x + 5 = 0$, differentiating yields:

$\displaystyle{\frac {dy}{dx} + \frac{dx}{dx} + \frac {d}{dx}5 = \frac {dy}{x} + 1 = 0}$.

Solving for $\frac{dy}{dx}$ yields:

$\displaystyle{\frac {dy}{dx} = -1}$

A circle can be described by the equation $x^2+y^2=r^2$ where $r$ is the radius of the circle. You can use implicit differentiation to find the slope of a line tangent to the circle at a point $(x,y)$. Since the slope of a tangent is the derivative at that point, we find the derivative implicitly:

$\displaystyle{2y \frac{dy}{dx} +2x \frac{dx}{dx}=\frac{dr}{dx}=0}$

where $\frac{dr}{dx}$ is $0$ since the radius is constant. You can then find a formula for $\frac{dy}{dx}$:

$\displaystyle{\frac{dy}{dx}=\frac{-2x}{2y}=\frac{-x}{y}}$

and you can now find the slope at any point $(x,y)$.

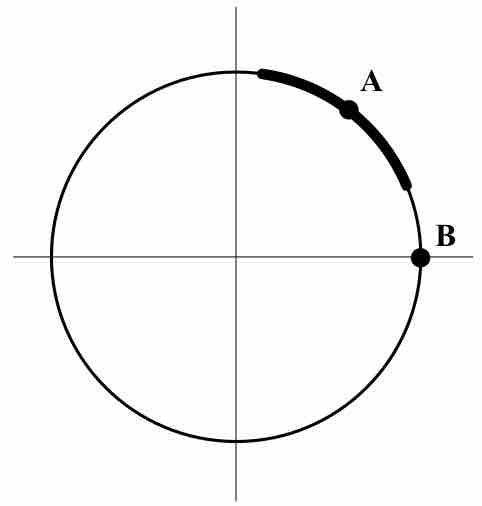
Path of a Point on a Circle

The path of a point on a circle can only be expressed as an implicit function.