Maximum and Minimum Values

In mathematics, the maximum and minimum (plural: maxima and minima) of a function, known collectively as extrema (singular: extremum), are the largest and smallest value that the function takes at a point either within a given neighborhood (local or relative extremum) or on the function domain in its entirety (global or absolute extremum).

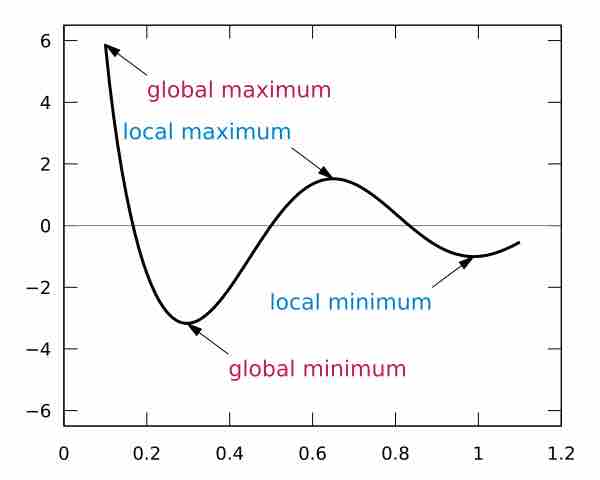
Maxima and Minima

Local and global maxima and minima for $\cos \frac{3πx}{x}$, $0.1 \leq x \leq 1.1$.

A real-valued function $f$ defined on a real line is said to have a local (or relative) maximum point at the point $x_{\text{max}}$, if there exists some $\varepsilon > 0$ such that $f(x_{\text{max}}) \geq f(x)$ when $\left | x - x_{\text{max}} \right | < \varepsilon$. The value of the function at this point is called maximum of the function. Similarly, a function has a local minimum point at $x_{\text{min}}$, if $f(x_{\text{min}}) \leq f(x)$ when $\left | x - x_{\text{min}} \right | < \varepsilon$. The value of the function at this point is called minimum of the function.

A function has a global (or absolute) maximum point at $x_{\text{MAX}}$ if $f(x_{\text{MAX}}) \geq f(x)$ for all $x$. Similarly, a function has a global (or absolute) minimum point at $x_{\text{MIN}}$ if $f(x_{\text{MIN}}) \leq f(x)$ for all $x$. The global maximum and global minimum points are also known as the arg max and arg min: the argument (input) at which the maximum (respectively, minimum) occurs.

Finding global maxima and minima is the goal of mathematical optimization. If a function is continuous on a closed interval, then by the extreme value theorem global maxima and minima exist. Furthermore, a global maximum (or minimum) either must be a local maximum (or minimum) in the interior of the domain, or must lie on the boundary of the domain. So a method of finding a global maximum (or minimum) is to look at all the local maxima (or minima) in the interior, and also look at the maxima (or minima) of the points on the boundary; and take the biggest (or smallest) one. Local extrema can be found by Fermat's theorem, which states that they must occur at critical points. One can distinguish whether a critical point is a local maximum or local minimum by using the first derivative test or second derivative test.