Real Numbers
Real numbers can be thought of as points on an infinitely long line called the number line or real line, where the points corresponding to integers are equally spaced. The real numbers include all the rational numbers, such as the integer -5 and the fraction
Any real number can be determined by a possibly infinite decimal representation such as that of 8.632, where each consecutive digit is measured in units one tenth the size of the previous one. The real line can be thought of as a part of the complex plane, and correspondingly, complex numbers include real numbers as a special case.
Functions
A function is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. An example is the function that relates each real number
Graphs
The graph of a function
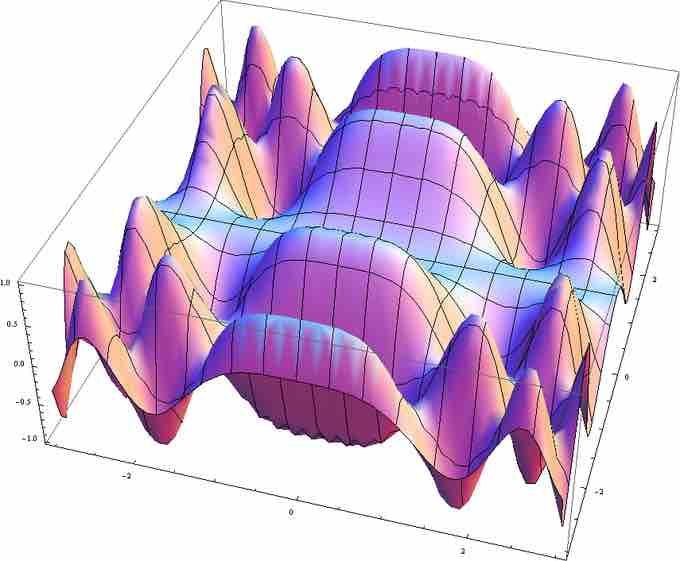
Graph of a Function
This is a graph of the function