A conservative vector field is a vector field which is the gradient of a function, known in this context as a scalar potential. Conservative vector fields have the following property: The line integral from one point to another is independent of the choice of path connecting the two points; it is path-independent.
Conversely, path independence is equivalent to the vector field's being conservative. Conservative vector fields are also irrotational, meaning that (in three dimensions) they have vanishing curl. In fact, an irrotational vector field is necessarily conservative provided that a certain condition on the geometry of the domain holds: it must be simply connected.
Definition: A vector field
For any scalar field
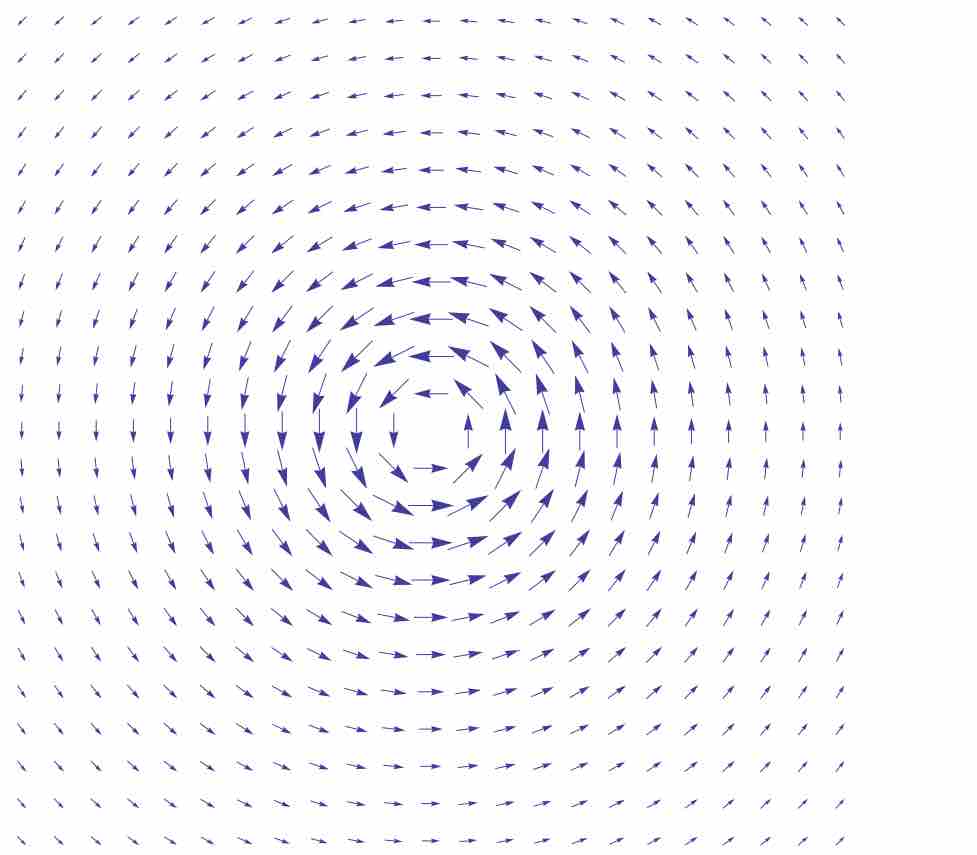
Fig 1
The above field
Path Independence
A key property of a conservative vector field is that its integral along a path depends only on the endpoints of that path, not the particular route taken. Suppose that

Line Integral Over Scalar Field
The line integral over a scalar field