Symmetry in Trigonometric Functions

We have previously discussed even and odd functions. Recall that even functions are symmetric about the $y$-axis, and odd functions are symmetric about the origin, $(0, 0)$. Recall that cosine is an even function because it is symmetric about the $y$-axis. On the other hand, sine and tangent are odd functions because they are symmetric about the origin. 

We will now consider each of the trigonometric functions and their cofunctions (secant, cosecant, and cotangent), and observe symmetry in their graphs. This symmetry is used to derive certain identities. 

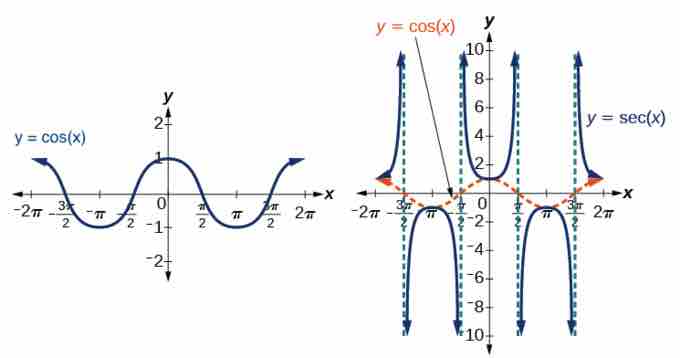
Symmetry around the $y$-axis

Cosine and secant are even functions, with symmetry around the $y$-axis.

The cosine and secant functions are symmetric about the y-axis. Graphs that are symmetric about the $y$-axis represent even functions. For even functions, any two points with opposite $x$-values have the same function value. This is expressed mathematically as $f(-x) = f(x)$ for all $x$ in the domain of $f$. 

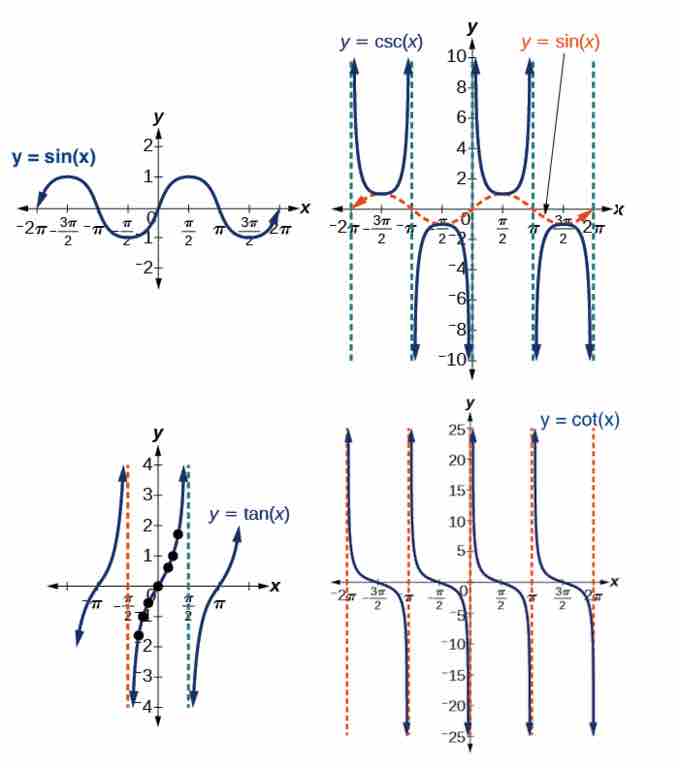
Symmetry around the origin

Sine, cosecant, tangent, and cotangent are odd functions, and are symmetric around the origin.

The sine, cosecant, tangent, and cotangent functions are symmetric about the origin. Graphs that are symmetric about the origin represent odd functions. For odd functions, any two points with opposite $x$-values also have opposite $y$-values. This is expressed mathematically as $f(-x) = -f(x)$ for all $x$ in the domain of $f$. 

Symmetry Identities 

We can apply the definitions for even and odd functions to derive symmetry identities that correspond to each of our six trigonometric functions. The following symmetry identities are useful in finding the trigonometric function of a negative value.

Notice that only two of the trigonometric identities are even functions: cosine and secant. For these functions, we apply $f(-x) = f(x)$ to find the following identities:

$\begin{aligned} \cos(-x) &= \cos x \\ \sec(-x) &= \sec x \end{aligned}$

For the odd trigonometric functions, we apply $f(-x) = -f(x)$ and find the following identities: 

$\begin{aligned} \sin(-x) &= - \sin x \\ \csc(-x) &= - \csc x \\ \tan(-x) &= - \tan x \\ \cot(-x) &= - \cot x \end{aligned}$

Example

Find the sine, cosine, and tangent of $\displaystyle{\theta = -\frac{5\pi}{6}}$.

First, we can identify that the absolute value of $\theta$ is a special angle, $\displaystyle{\frac{5\pi}{6}}$. We know from the unit circle that $\displaystyle{\cos{\left(\frac{5\pi}{6}\right)} = -\frac{\sqrt{3}}{2} }$ and $\displaystyle{\sin{\left(\frac{5\pi}{6}\right)} = \frac{1}{2} }$. 

Using these values from the unit circle, we can calculate $\displaystyle{\tan{\left(\frac{5\pi}{6}\right)}}$:

$\displaystyle{ \begin{aligned} \tan{\left(\frac{5\pi}{6}\right)} &= \frac{\sin{\left(\frac{5\pi}{6}\right)}}{\cos{\left(\frac{5\pi}{6}\right)}} \\ &= \frac{\frac{1}{2}}{-\frac{\sqrt{3}}{2}} \\ &= \left(\frac{1}{2}\right) \cdot \left(-\frac{2}{\sqrt{3}}\right) \\ &= -\frac{1}{\sqrt{3}} \end{aligned} }$

Now that we know the sine, cosine, and tangent of $\displaystyle{\frac{5\pi}{6}}$, we can apply the symmetry identities to find the functions of $\displaystyle{-\frac{5\pi}{6}}$. 

Applying the symmetry identity for cosine, we have:

 $\displaystyle{ \begin{aligned} \cos{\left(-\frac{5\pi}{6}\right)} &= \cos{\left(\frac{5\pi}{6}\right)} \\ &= -\frac{\sqrt{3}}{2} \end{aligned} }$

Applying the identity for sine, we have:

$\displaystyle{ \begin{aligned} \sin{\left(-\frac{5\pi}{6}\right)} &= - \sin{\left(\frac{5\pi}{6}\right)} \\ &= - \frac{1}{2} \end{aligned} }$ 

Finally, applying the identity for tangent, we have:

$\displaystyle{ \begin{aligned} \tan{\left(-\frac{5\pi}{6}\right)} &= - \tan{\left(\frac{5\pi}{6}\right)} \\ &= -\left(-\frac{1}{\sqrt{3}}\right) \\ &= \frac{1}{\sqrt{3}} \end{aligned} }$