Types of Asymptotes
In analytic geometry, an asymptote of a curve is a line such that the distance between the curve and the line approaches zero as they tend to infinity.
There are three kinds of asymptotes: horizontal, vertical and oblique. Horizontal asymptotes of curves are horizontal lines that the graph of the function approaches as
Vertical asymptotes are vertical lines near which the function grows without bound. They are parallel to the
An asymptote that is neither horizontal or vertical is an oblique (or slant) asymptote. These are diagonal lines so that the difference between the curve and the line approaches
Each type of asymptote is shown in the graph below.
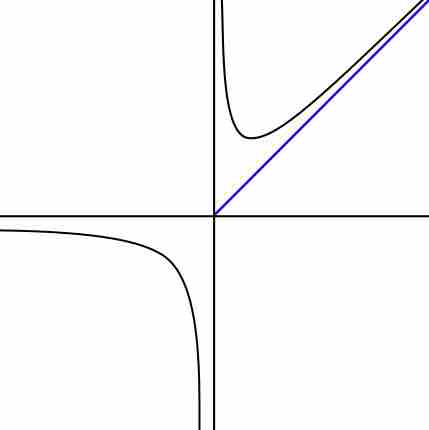
Graph with asymptotes
The graph of a function with a horizontal (
Example 1
Consider the graph of the equation

Graph of $f(x) = 1/x$
Both the
Notice that as the positive values of
Similarly, as the positive values of
Asymptotes of Rational Functions
A rational function has at most one horizontal or oblique asymptote, and possibly many vertical asymptotes.
Vertical asymptotes occur only when the denominator is zero. In other words, vertical asymptotes occur at singularities, or points at which the rational function is not defined. Vertical asymptotes only occur at singularities when the associated linear factor in the denominator remains after cancellation.
For example, consider the function:
We can identify from the linear factors in the denominator that two singularities exist, at
The degree of the numerator and degree of the denominator determine whether or not there are any horizontal or oblique asymptotes.
Existence of horizontal asymptote depends on the degree of polynomial in
the numerator (
-
If
$n>m$ , then there is no horizontal asymptote (However, if$n = m+1$ , then there exists a slant asymptote). -
If
$n<m$ , then the$x$ -axis is a horizontal asymptote. -
If
$n=m$ , then a horizontal asymptote exists, and the equation is:
When the numerator of a rational function has degree exactly one greater than the denominator, the function has an oblique (slant) asymptote. The asymptote is the polynomial term after dividing the numerator and denominator, and is a linear expression.
Example 2
Find any vertical asymptotes of
Notice that, based on the linear factors in the denominator, singularities exists at
Example 3
Find any horizontal or oblique asymptote of
Because the polynomials in the numerator and denominator have the same degree (
The coefficient of the highest power term is