The Rayleigh Criterion

The Rayleigh criterion determines the separation angle between two light sources which are distinguishable from each other.

Learning Objective

Explain meaning of the Rayleigh criterion

Key Points

Diffraction plays a large part in the resolution at which we are able to see things. There is a point where two light sources can be so close to each other that we cannot distinguish them apart.
When two light sources are close to each other, they can be: unresolved (i.e., not able to distinguish one from the other), just resolved (i.e., only able to distinguish them apart from each other), and a little well resolved (i.e., easy to tell apart from one another).
In order for two light sources to be just resolved, the center of one diffraction pattern must directly overlap with the first minimum of the other diffraction pattern.

Terms

resolution
The degree of fineness with which an image can be recorded or produced, often expressed as the number of pixels per unit of length (typically an inch).
diffraction
The bending of a wave around the edges of an opening or an obstacle.

Example

Calculate the minimum angular spreading of a flashlight beam that is originally 5.00 cm in diameter with an average wavelength of 600 nm. We are given: D - 5 cmλ - 600 nm. We are looking for: θ$\theta = 1.22 \frac{\lambda}D\\\theta = 1.22 \frac{600 nm}{5 cm}\\\theta = 1.46*10^{-5} rad$

Full Text

Resolution Limits

Along with the diffraction effects that we have discussed in previous atoms, diffraction also limits the detail that we can obtain in images. shows three different circumstances of resolution limits due to diffraction:

Resolution Limits

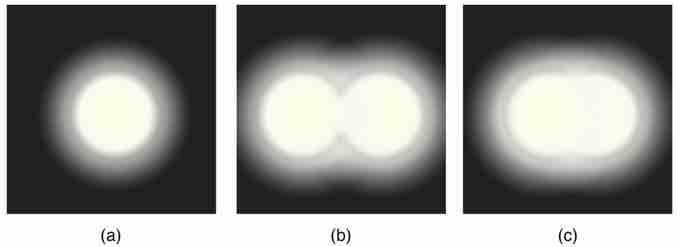
(a) Monochromatic light passed through a small circular aperture produces this diffraction pattern. (b) Two point light sources that are close to one another produce overlapping images because of diffraction. (c) If they are closer together, they cannot be resolved or distinguished.

(a) shows a light passing through a small circular aperture. You do not see a sharp circular outline, but a spot with fuzzy edges. This is due to diffraction similar to that through a single slit.
(b) shows two point sources close together, producing overlapping images. Due to the diffraction, you can just barely distinguish between the two point sources.
(c) shows two point sources which are so close together that you can no longer distinguish between them.

This effect can be seen with light passing through small apertures or larger apertures. This same effect happens when light passes through our pupils, and this is why the human eye has limited acuity.

Rayleigh Criterion

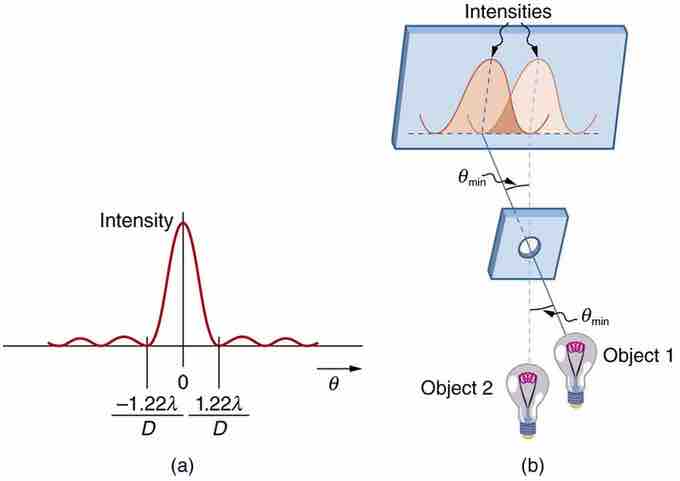
In the 19th century, Lord Rayleigh invented a criteria for determining when two light sources were distinguishable from each other, or resolved. According to the criteria, two point sources are considered just resolved (just distinguishable enough from each other to recognize two sources) if the center of the diffraction pattern of one is directly overlapped by the first minimum of the diffraction pattern of the other. If the distance is greater between these points, the sources are well resolved (i.e., they are easy to distingiush from each other). If the distance is smaller, they are not resolved (i.e., they cannot be distinguished from each other). The equation to determine this is:

$\theta = 1.22 \frac{\lambda}D$

θ - angle the objects are separated by, in radian λ - wavelength of light D - aperture diameter. shows this concept visually. This equation also gives the angular spreading of a source of light having a diameter D.

Rayleigh Criterion

(a) This is a graph of intensity of the diffraction pattern for a circular aperture. Note that, similar to a single slit, the central maximum is wider and brighter than those to the sides. (b) Two point objects produce overlapping diffraction patterns. Shown here is the Rayleigh criterion for being just resolvable. The central maximum of one pattern lies on the first minimum of the other.

[ edit ]

Prev Concept

Single Slit Diffraction

Thin Film Interference

Next Concept