Below is an illustration of pair production, which refers to the creation of an elementary particle and its antiparticle, usually when a photon interacts with a nucleus. For example, an electron and its antiparticle, the positron, may be created. This is allowed, provided there is enough energy available to create the pair (i.e., the total rest mass energy of the two particles) and that the situation allows both energy and momentum to be conserved. Some other conserved quantum numbers such as angular momentum, electric charge, etc., must sum to zero as well. The probability of pair production in photon-matter interactions increases with increasing photon energy, and also increases with atomic number ($Z$) of the nucleus approximately as $Z^2$.

Pair Production

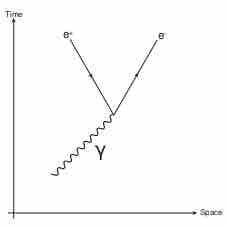
Feynman diagram for pair production. A photon decays into an electron-positron pair.

$\gamma + \gamma \rightarrow e^{-} + e^{+}$

In nuclear physics, this reaction occurs when a high-energy photon (gamma rays) interacts with a nucleus. The energy of this photon can be converted into mass through Einstein's equation $E=mc^2$ where $E$ is energy, $m$ is mass and $c$ is the speed of light. The photon must have enough energy to create the mass of an electron plus a positron. The mass of an electron is $9.11 \cdot 10^{-31}$ kg (equivalent to 0.511 MeV in energy), the same as a positron.

Without a nucleus to absorb momentum, a photon decaying into electron-positron pair (or other pairs for that matter) can never conserve energy and momentum simultaneously. The nucleus in the process carries away (or provides) access momentum.

The reverse process is also possible. The electron and positron can annihilate and produce two 0.511 MeV gamma photons. If all three gamma rays, the original with its energy reduced by 1.022 MeV and the two annihilation gamma rays, are detected simultaneously, then a full energy peak is observed.

These interactions were first observed in Patrick Blackett's counter-controlled cloud chamber, leading him to receive the 1948 Nobel Prize in Physics.