Balancing Nuclear Equations

Nuclear reactions may be shown in a form similar to chemical equations, for which invariant mass, which is the mass not considering the mass defect, must balance for each side of the equation. The transformations of particles must follow certain conservation laws, such as conservation of charge and baryon number, which is the total atomic mass number. An example of this notation follows:

$^6_3Li\ +\ \ ^2_1H\rightarrow \ ^4_2He\ + \ ?$

To balance the equation above for mass, charge, and mass number, the second nucleus on the right side must have atomic number 2 and mass number 4; it is therefore also helium-4. The complete equation therefore reads:

$^6_3Li\ +\ \ ^2_1H\rightarrow \ ^4_2He\ \ +\ \ ^4_2He$

Or, more simply:

$^6_3Li\ +\ \ ^2_1H\rightarrow 2\ ^4_2He$

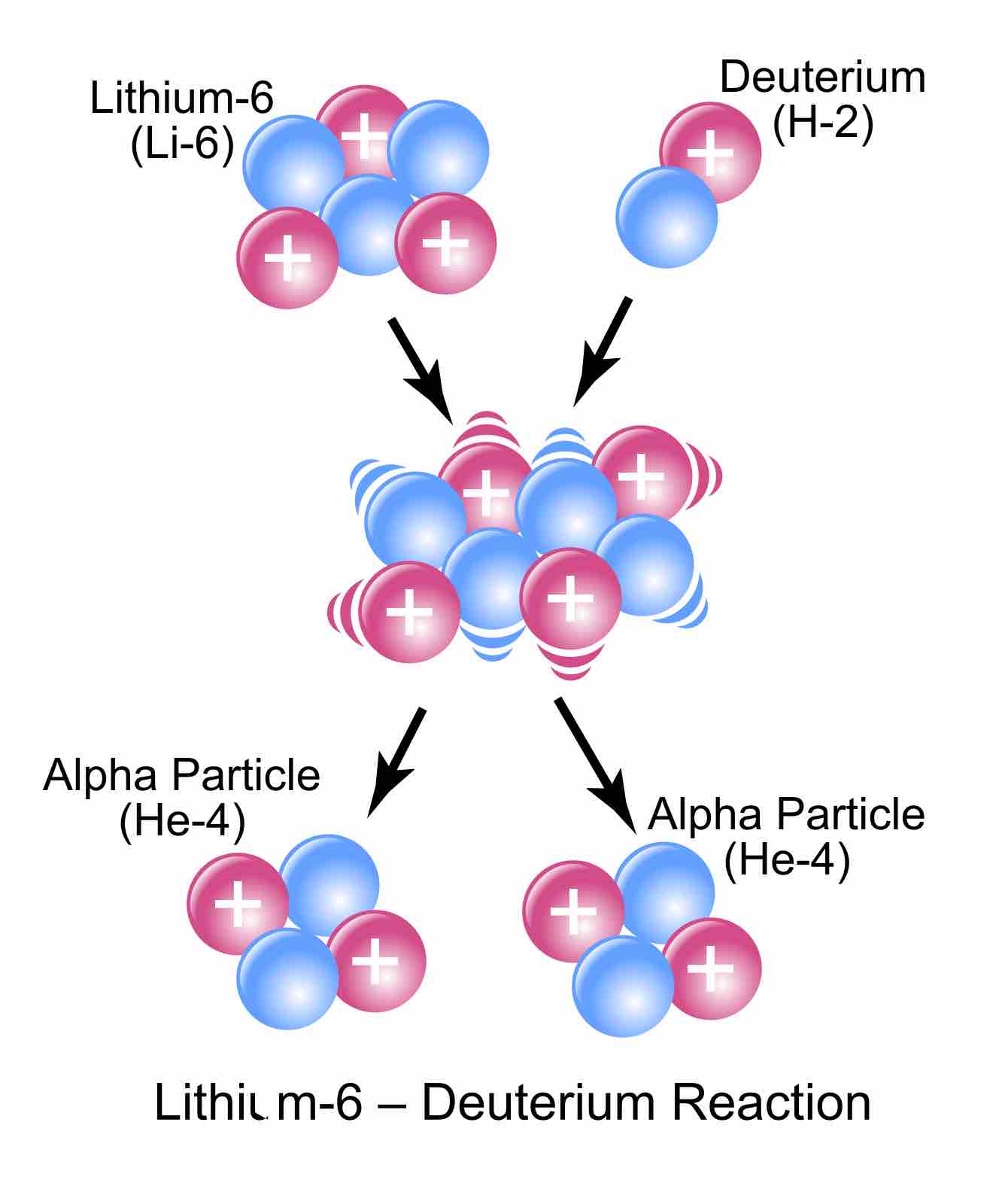
Lithium-6 plus deuterium gives two helium-4s.

The visual representation of the equation we used as an example.

Compact Notation of Radioactive Decay

Instead of using the full equations in the style above, in many situations a compact notation is used to describe nuclear reactions. This style is of the form A(b,c)D, which is equivalent to A + b gives c + D. Common light particles are often abbreviated in this shorthand, typically p for proton, n for neutron, d for deuteron, α representing an alpha particle or helium-4, β for beta particle or electron, γ for gamma photon, etc. The reaction in our example above would be written as Li-6(d,α)α.

Balancing a Radioactive Decay Equation

In balancing a nuclear equation, it is important to remember that the sum of all the mass numbers and atomic numbers, given on the upper left and lower left side of the element symbol, respectively, must be equal for both sides of the equation. In addition, problems will also often be given as word problems, so it is useful to know the various names of radioactively emitted particles.

Example

$^{ 235 }_{ 92 }U \rightarrow \ \ ^{ 231 }_{ 90 }Th\ +\ \ ?$

This could be written out as uranium-235 gives thorium-231 plus what? In order to solve, we find the difference between the atomic masses and atomic numbers in the reactant and product. The result is an atomic mass difference of 4 and an atomic number difference of 2. This fits the description of an alpha particle. Thus, we arrive at our answer:

$^{ 235 }_{ 92 }U \rightarrow \ \ ^{ 231 }_{ 90}Th + \ \ ^{ 4 }_{ 2 }He$

Example

$^{ 214 }_{ 84 }Po\ +\ \ 2\ ^{ 4 }_{ 2 }He\ +\ 2^0_{-1}e\rightarrow \ \ \ ?$

This could also be written out as polonium-214, plus two alpha particles, plus two electrons, give what? In order to solve this equation, we simply add the mass numbers, 214 for polonium, plus 8 (two times four) for helium (two alpha particles), plus zero for the electrons, to give a mass number of 222. For the atomic number, we take 84 for polonium, add 4 (two times two) for helium, then subtract two (two times -1) for two electrons lost through beta emission, to give 86; this is the atomic number for radon (Rn). Therefore, the equation should read:

$^{ 214 }_{ 84 }Po+2^{ 4 }_{ 2 }He+2^0_{-1}e\rightarrow\ \ ^{222}_{86}Rn$