The reaction quotient, Q, is a measure of the relative amounts of reactants and products during a chemical reaction at a given point in time. By comparing the value of Q to the equilibrium constant, Keq, for the reaction, we can determine whether the forward reaction or reverse reaction will be favored. Take the following generic equation:
The reaction quotient, Q, takes the following form:
Note that the reaction quotient takes the exact same form as the equilibrium constant, and is a function of concentrations and/or activities of the reactants and products. The difference is that Q applies when the reaction is at non-equilibrium conditions, and therefore its value can vary. Just as for the equilibrium constant, the reaction quotient can be a function of activities or concentrations.
The reaction quotient can be used to determine whether a reaction under specified conditions will proceed spontaneously in the forward direction or in the reverse direction. Three properties can be derived from this definition of the reaction quotient:
- If Q = Keq, the reaction is at equilibrium.
- If Q < Keq, the reaction will move to the right (in the forward direction) in order to reach equilibrium.
- If Q > Keq, the reaction will move to the left (in the reverse direction) in order to reach equilibrium.
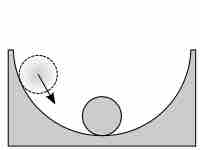
Moving toward equilibrium
The ball in the initial state is indicative a reaction in which Q < K; in order to reach equilibrium conditions, the reaction proceeds forward.
As the reaction proceeds, assuming that there is no energy barrier, the species' concentrations, and hence the reaction quotient, change. Eventually, the concentrations become constant; at this point, the reaction is at equilibrium. The equilibrium constant, Keq, can be expressed as follows:
This expression shows that Q will eventually become equal to Keq, given an infinite amount of time. However, most reactions will generally reach equilibrium in a finite period of time.