Calculating Equilibrium Concentrations

The ICE Chart

The theory of chemical equilibrium tells us that the species involved in a reversible reaction will eventually arrive at constant concentrations. Let's consider the following reaction:

$N_2 + O_2 \rightleftharpoons 2NO$

Initially, the concentration for each species is as follows: [N₂]₀ = [O₂]₀ = 0.1 M, and [NO]₀ = 0 M. The value of Kc for this reaction is known to be 0.1 (we will assume that this experimentally determined quantity  is given to us). We can write the following K_c expression based on this starting information:

$K_{c} = \frac{[NO]^{2}}{[N_{2}][O_{2}]}=0.1$

Since we know what our K_c value  is and the initial concentrations of reactants, we can set up an ICE chart to track the changes in concentration, as the reaction proceeds towards equilibrium. ICE stands for "initial, change, equilibrium."

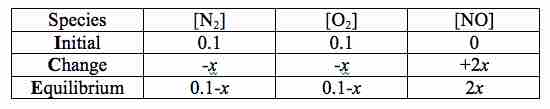
ICE chart for the reaction of nitrogen and oxygen to form nitric oxide

The equilibrium concentration is the sum of the initial concentration and the change, which is derived from the reaction stoichiometry.

Note that the values of x in the third row of our chart indicate the change in concentration of each species over the course of the reaction. The coefficients present in the balanced equation tell us how many moles of atoms or molecules participate in the reaction. Using these coefficients, the balanced equation tells us that for every mole of N₂ and mole O₂ consumed, 2 moles of NO are produced. We can designate x as the change in concentration of N₂ and O_2. As reactants located on the left hand side of our balanced equation, the sign is negative as they are being consumed.  Similarly, we designate +2x as the change in concentration for NO, but it's positive because it's being produced. After we fill in our chart we can determine the equilibrium concentrations by adding down the columns of the ICE chart. The equilibrium concentrations for each species are therefore: [N₂] = 0.1 - x; [O₂] = 0.1 - x; [NO] = 2x.

Plugging into the K_C Expression and Solving for x

Now that we have expressions for the equilibrium concentrations of each species, we can substitute them into our expression for K_c:

$K_{c} = \frac{[NO]^{2}}{[N_{2}][O_{2}]}=\frac{(2x)^{2}}{(0.1 - x)(0.1 - x)}$

If we expand, collect terms, and solve for x, we get the following quadratic equation:

$x\quad =\quad \frac {{-0.2}{ { { K }_{ c } }{ \pm }{ \sqrt { \left( .2{ K }_{ c } \right) ^{ 2 }{ -4\left( 4-{ K }_{ c } \right) \left( -0.01{ K }_{ c } \right) } } } } }{ { 2 }\left( 4-{ K }_{ c } \right) }$

When solving a quadratic equation, we will always get two values for x. The two x values are -0.1188M and 0.0137M. Only one of these values involves equilibrium concentrations that are actually possible. We can determine which x value is the real solution by substituting it into our equilibrium concentrations, found on the ICE chart. For example, consider the value x = −0.0188. Substituting this into the equilibrium amount for N₂ gives a concentration of 0.1 - (-0.0188) = 0.1188 M. This is clearly impossible, since we cannot have more N₂ at equilibrium than we had at the beginning. Therefore, we use the other root for x,which is 0.0137.

Knowing the initial concentration values and equilibrium constant we were able to calculate the equilibrium concentrations for N₂, O₂ and NO. In the system we evaluated, at equilibrium we would expect to find that [O₂]_eq = [N₂]_eq = 0.086 M and [NO]_eq = 0.028 M. Note that we could have solved for the amount of NO produced rather than for the amount of N₂ and O₂ consumed. The result would be the same.