Definition of Inverse Function

An inverse function, which is notated $f^{-1}(x) $, is defined as the inverse function of $f(x)$ if it consistently reverses the $f(x)$ process. That is, if $f(x)$ turns $a$ into $b$, then $f^{-1}(x)$ must turn $b$ into $a$.  More concisely and formally, $f^{-1}(x)$ is the inverse function of $f(x)$ if:

$f({f}^{-1}(x))=x$

Below is a mapping of function $f(x)$  and its inverse function, $f^{-1}(x)$.  Notice that the ordered pairs are reversed from the original function to its inverse.  Because $f(x)$ maps $a$ to $3$, the inverse $f^{-1}(x)$ maps $3$ back to $a$.

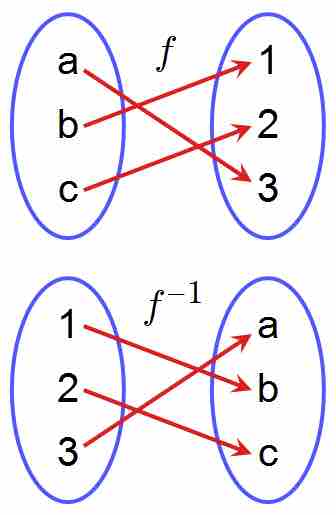
Inverse functions: mapping representation

An inverse function reverses the inputs and outputs.

Thus the graph of $f^{-1}(x)$ can be obtained from the graph of $f(x)$ by switching the positions of the $x$ and $y$-axes. This is equivalent to reflecting the graph across the line $y=x$, an increasing diagonal line through the origin.

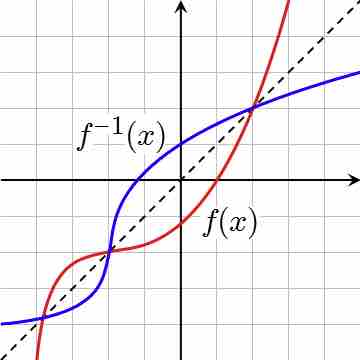
Inverse functions: graphic representation

The function graph (red) and its inverse function graph (blue) are reflected about the line $y=x$ (dotted black line). Notice that any ordered pair on the red curve has its reversed ordered pair on the blue line. For example, $(0,1)$ on the red (function) curve is reflected over the line $y=x$ and becomes $(1,0)$ on the blue (inverse function) curve. 

Write the Inverse Function 

In general, given a function, how do you find its inverse function? Remember that an inverse function reverses the inputs and outputs.  So to find the inverse function, switch the $x$ and $y$ values of a given function, and then solve for $y$.

Example 1  

Find the inverse of : $f(x)=x^2$

a. : Write the function as:  $y=x^2$

b. : Switch the $x$ and $y$ variables: $x=y^2$

c. : Solve for $y$:

 $\begin {aligned} x&=y^2 \\ \pm\sqrt{x}&=y \end {aligned}$

Since the function $f(x)=x^2$ has multiple outputs, its inverse is not a function. Notice the graphs in the picture below.  Even though the blue curve is a function (passes the vertical line test), its inverse would not be. The red curve for the function $f(x)=\sqrt{x}$ is not the full inverse of the function $f(x)=x^2$

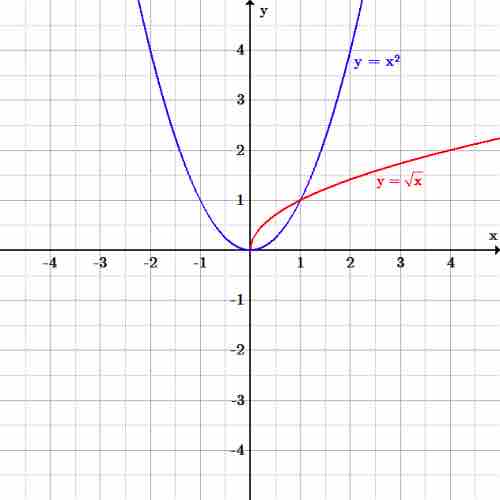
The inverse is not a function

A function's inverse may not always be a function.  The function (blue) $f(x)=x^2$, includes the points $(-1,1)$ and $(1,1)$.  Therefore, the inverse would include the points: $(1,-1)$ and $(1,1)$ which the input value repeats, and therefore is not a function. For $f(x)=\sqrt{x}$ to be a function, it must be defined as positive.

Example 2 

Find the inverse function of : $f(x)=2^x$

As soon as the problem includes an exponential function, we know that the logarithm reverses exponentiation.  The complex logarithm is the inverse function of the exponential function applied to complex numbers.  Let's see what happens when we switch the input and output values and solve for $y$.

a. : Write the function as: $y = {2}^{x}$

b. : Switch the $x$ and $y$ variables: $x = {2}^{y}$

c. : Solve for $y$: 

 $\begin {aligned} {log}_{2}x &= {log}_{2}{2}^{y} \\{log}_{2}x &= y{log}_{2}{2} \\{log}_{2}x &= y \\{f}^{1}(x) &= {log}_{2}(x) \end {aligned}$

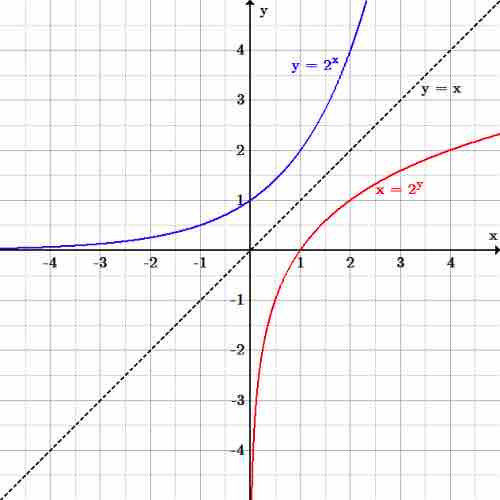
Exponential and logarithm functions

The graphs of $y=2^x$ (blue) and $x=2^y$ (red) are inverses of one another. The black line represents the line of reflection, in which is $y=x$. 

Test to make sure this solution fills the definition of an inverse function.

1. Pick a number, and plug it into the original function. $2\rightarrow f(x)\rightarrow 4$.
2. See if the inverse function reverses this process. $4\rightarrow f^{-1}(x)\rightarrow 2$. ✓