The definition of a circle is as simple as the shape. A circle is the set of all points that are at a certain distance from a center point. This definition is what gives us the concept of the radius of a circle, which is equal to that certain distance.
Since we know a circle is the set of points a fixed distance from a center point, let's look at how we can construct a circle in a Cartesian coordinate plane with variables
If we now square this equation on both sides, we have:
Remember that the distance between the center
This is the general formula for a circle with center
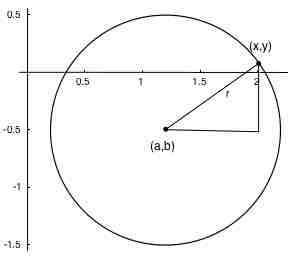
Graph of a circle
The circle with center
Parts of a Circle
Now that we have an algebraic foundation for the circle, let's connect it to what we already know about some different parts of the circle.
Diameter
The diameter is any straight line that passes through the center of the circle. It is equal to twice the radius, so:
Circumference
The circumference is the length of the path around the circle. Algebraically it is given by:
or equivalently by
Area
The area of a circle is given by:
Notice that the radius is the only defining parameter for the size of any particular circle, and so it is the only variable that the area depends on.